Question

How do you find the amplitude, period, and shift for `y =3cos(6x+8pi)`?

Answer 1

See solution below.

Explanation:

Amplitude:

In a function of the form `y = acosb(x + c) + d`, the amplitude is `| a |`. Therefore, your amplitude is of 3.

Phase shift:

The phase shift can be found by setting the numbers in parentheses to 0.

`6x + 8pi = 0`

`6x = -8pi`

`x = (-4pi)/3`

Therefore, the phase shift is `(4pi)/3` units left.

Period:

The formula for period in sine and cosine functions is `P = (2pi)/b`, where P is the period and b is term b in your equation.

In your equation, we're going to have to factor b out.

`y = 3cos(6x + 8pi) -> y = 3cos2(3x + 4pi) -> b = 2`

`P = (2pi)/2`

`P = pi`

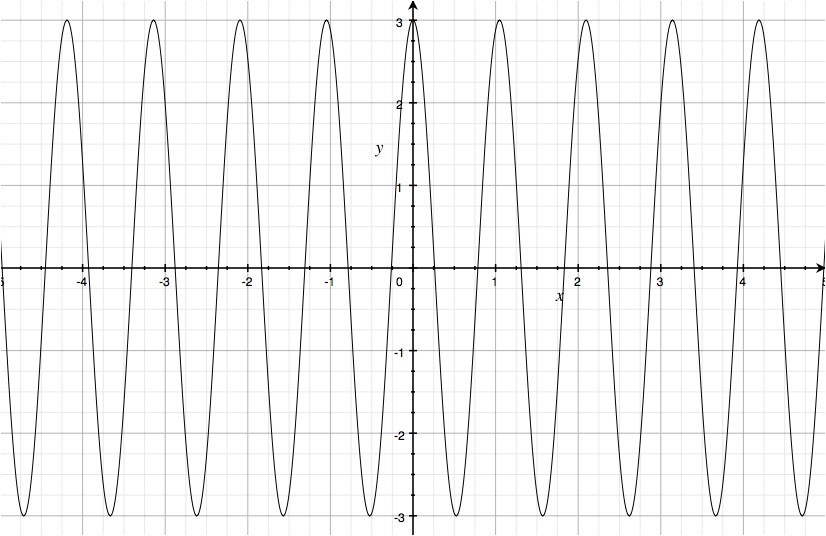
Here is the graph of your function:

Practice exercises:

1. Identify the period, phase shift and amplitude of `y = -4cos(2x - pi/2)`. Graph if possible.

Good luck!