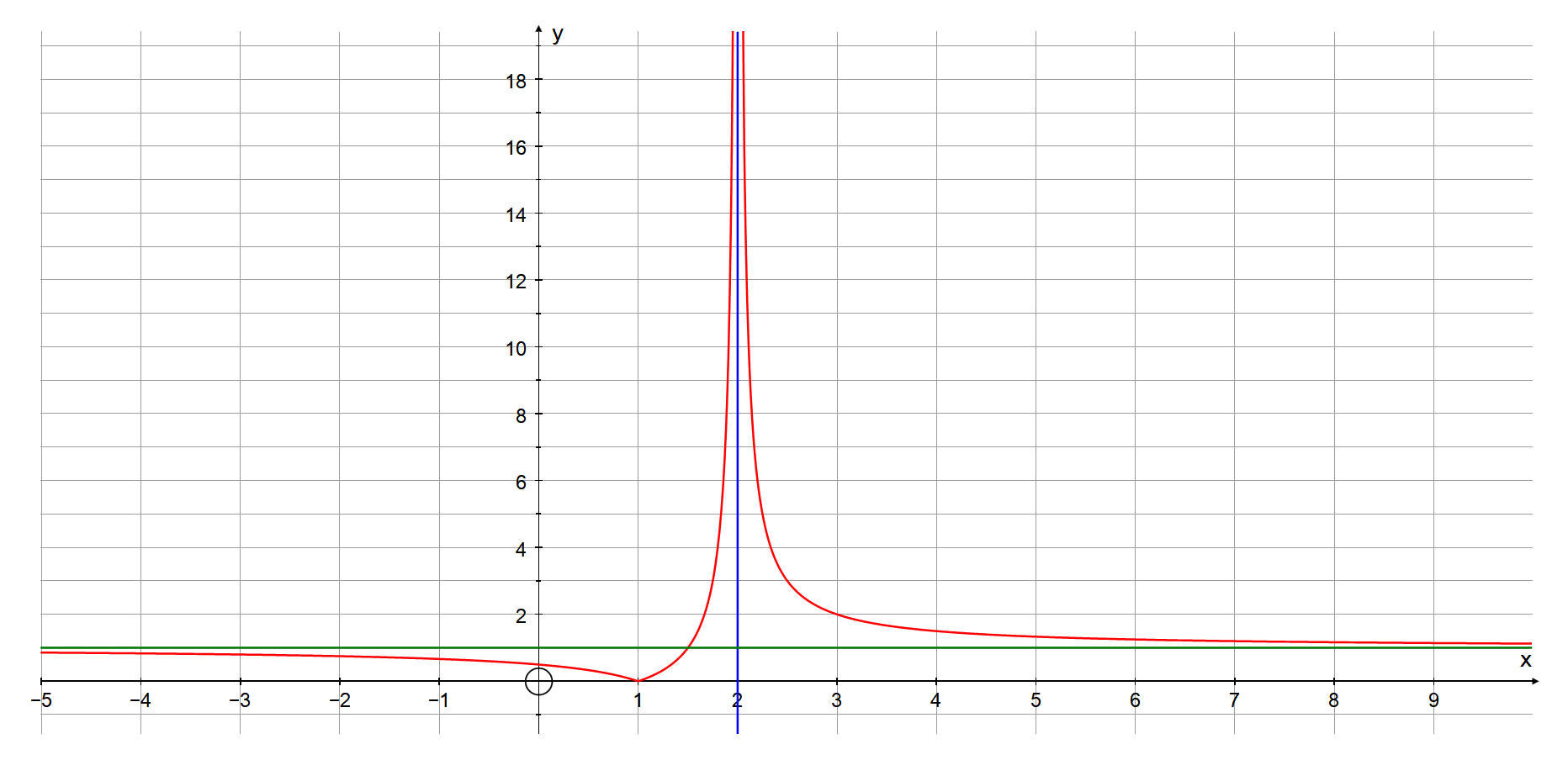
How do you find the asymptotes of #y=|x-1|/|x-2|#?

Question

2736 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-03-30T18:35:04

#color(blue)(x=2)#

#color(blue)(y=1)#

By definition of absolute value:

#y=(x-1)/(x-2)# and #y=(-(x-1))/(-(x-2))#

So these are both

#y=(x-1)/(x-2)#

This is undefined for #x=2#, so,

#color(blue)(x=2) \ \ \ \ \ \ # is a vertical asymptote.

We now check end behaviour:

For limits to infinity we only need be concerned with the highest powers of #x#, so:

#(x-1)/(x-2)->x/x=1#

as #x->oo#, # \ \ \ \ \ \ \ \ \ \ \ \ \ (x-1)/(x-2)->1#

as #x->-oo#, # \ \ \ \ \ \ \ \ \(x-1)/(x-2)->1#

So the line:

#color(blue)(y=1) \ \ \ \ # is a horizontal asymptote

GRAPH:

enter image source here