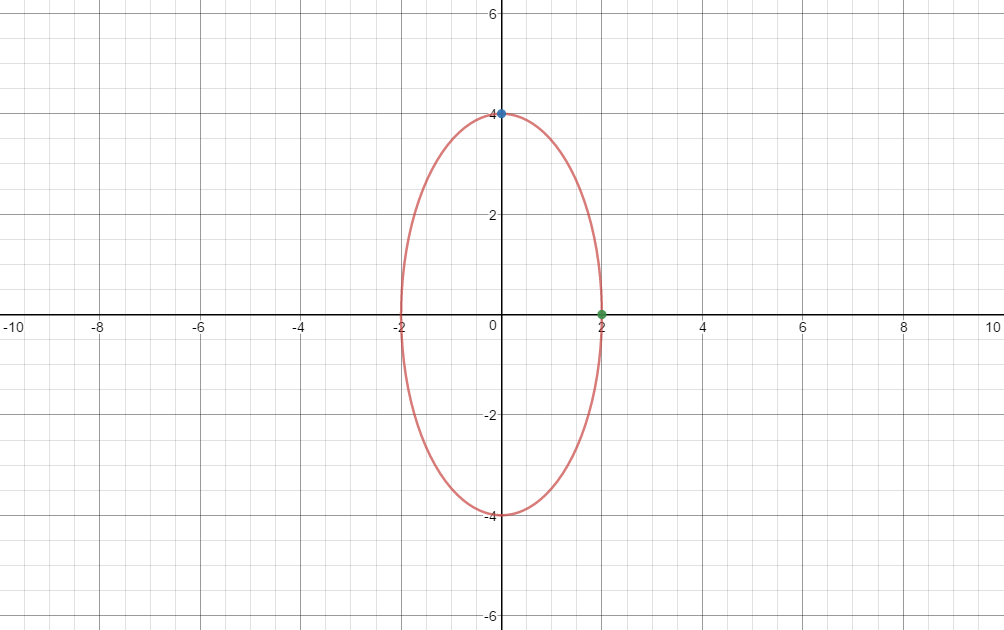
How do you find the equation of an ellipse with major axis vertical and passes through points (0,4) and (2,0)?
1 Answer
This problem can only be done, if one assumes that two given points,
Explanation:
The general Cartesian equation for an ellipse with a vertical major axis is:
Where x and y correspond any point,
Using the previously stated assumption.
The upper vertex on the major axis will have the coordinates:
The right vertex on the minor axis will have the coordinates:
Matching these two point to the given points:
This allows us to write the following equations:
Substituting 0 for h and k:
Substitute these 4 values into equation [1]:
Equation [2] is the answer.
Here is a graph with the ellipse and the two points plotted: