Question

How do you fInd the equation(s) of the tangent line(s) at the points) on the graph of the equation `y^2 -xy-10=0`, where x=3?

Answer 1

Please see the explanation.

Explanation:

When x = 3, the equation becomes:

`y^2 - 3y - 10 = 0`

This factors into:

`(y + 2)(y - 5) = 0`

The roots are:

`y = -2 and y = 5`

The points of tangency are `(3, -2) and (3, 5)`

Now, use implicit differentiation to compute the first derivative.

`2ydy/dx - y - xdy/dx = 0`

`(2y - x)dy/dx = y`

`dy/dx = y/(2y -x)`

At the point `(3, -2)`, the slope of the tangent line is:

`m = (-2)/(2(-2) - 3) = 2/7`

`-2 = 2/7(3) + b`

`b = -20/7`

The equation of the tangent line is:

`y = 2/7x - 20/7`

At the point `(3, 5)`, the slope of the tangent line is:

`m = 5/(2(5) - 3) = 5/7`

`5 = 5/7(3) + b`

`b = 20/7`

The equation of the tangent line is:

`y = 5/7x + 20/7`

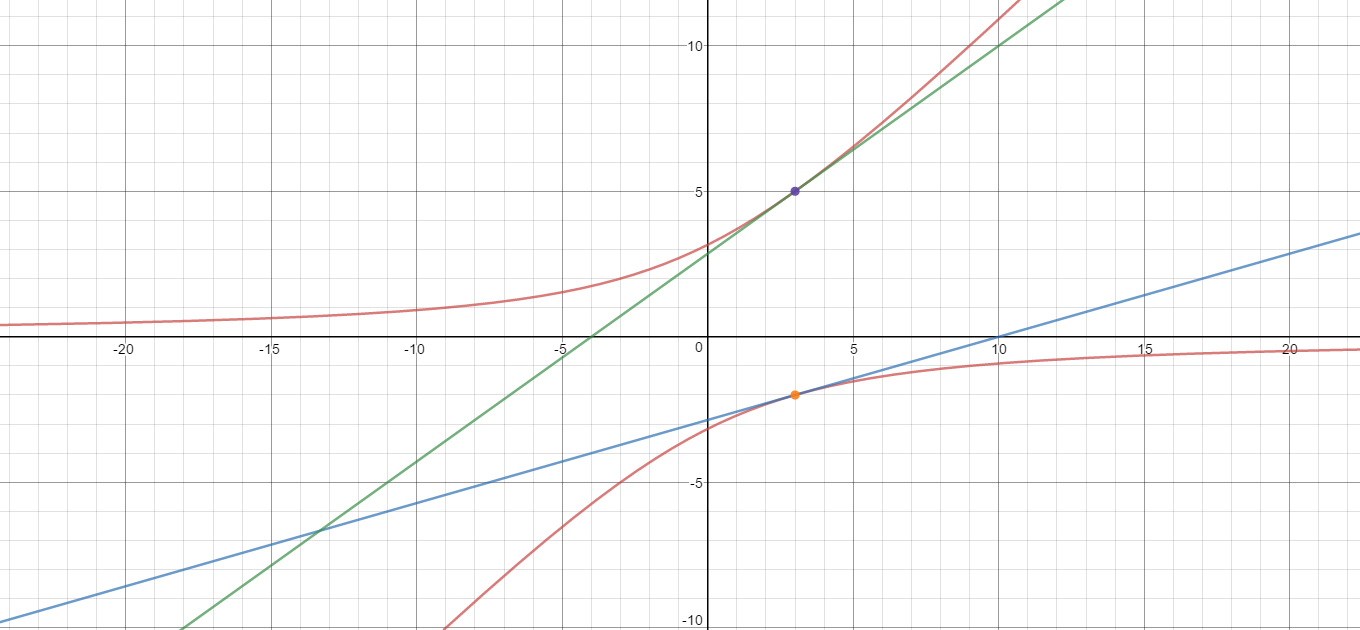
The following graph shows the curve, the points of tangency, and the tangent lines.