Question

How do you find the exact value of `cos( 2 arctan (5/12) )`?

Answer 1

`119/169`

Explanation:

Use the cosine double angle formula:

`cos(2x)=2cos^2(x)-1`

Here, inside the cosine function, the angle `arctan(5/12)` is being doubled, so we see that

`cos(2arctan(5/12))=2cos^2(arctan(5/12))-1`

To find `cos(arctan(5/12))`, draw a picture where `tan(beta)=5/12`, or where `5` is the opposite side's length and `12` is the adjacent side's length.

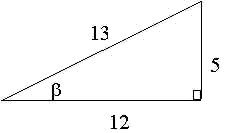
In this triangle, `13` is the hypotenuse (through the Pythagorean Theorem), and `cos(beta)=12/13`. This also means that `cos(arctan(5/12))=12/13`.

So we see that

`2cos^2(arctan(5/12))-1=2(12/13)^2-1=288/169-1=119/169`