Question

How do you find the length of `a` and `b` of a right triangle using angles of `A` and `B` and the distance from `c` to `C`? The angles of `A` and `B` are `45` degrees with `C` being `90` degrees and the distance from `C` to the hypotenuse (`c`) is `22^"`.

Answer 1

This is an isosceles triangle since the opposite angles of `a` and `b` are the same, so the lengths of `a` and `b` will be the same: `31.1` inches.

Explanation:

Here's a diagram: I always encourage people to draw a diagram. I drew it without a protractor or set square, using the side of my calculator for a ruler, so it's not to scale, but it is helpful anyway.

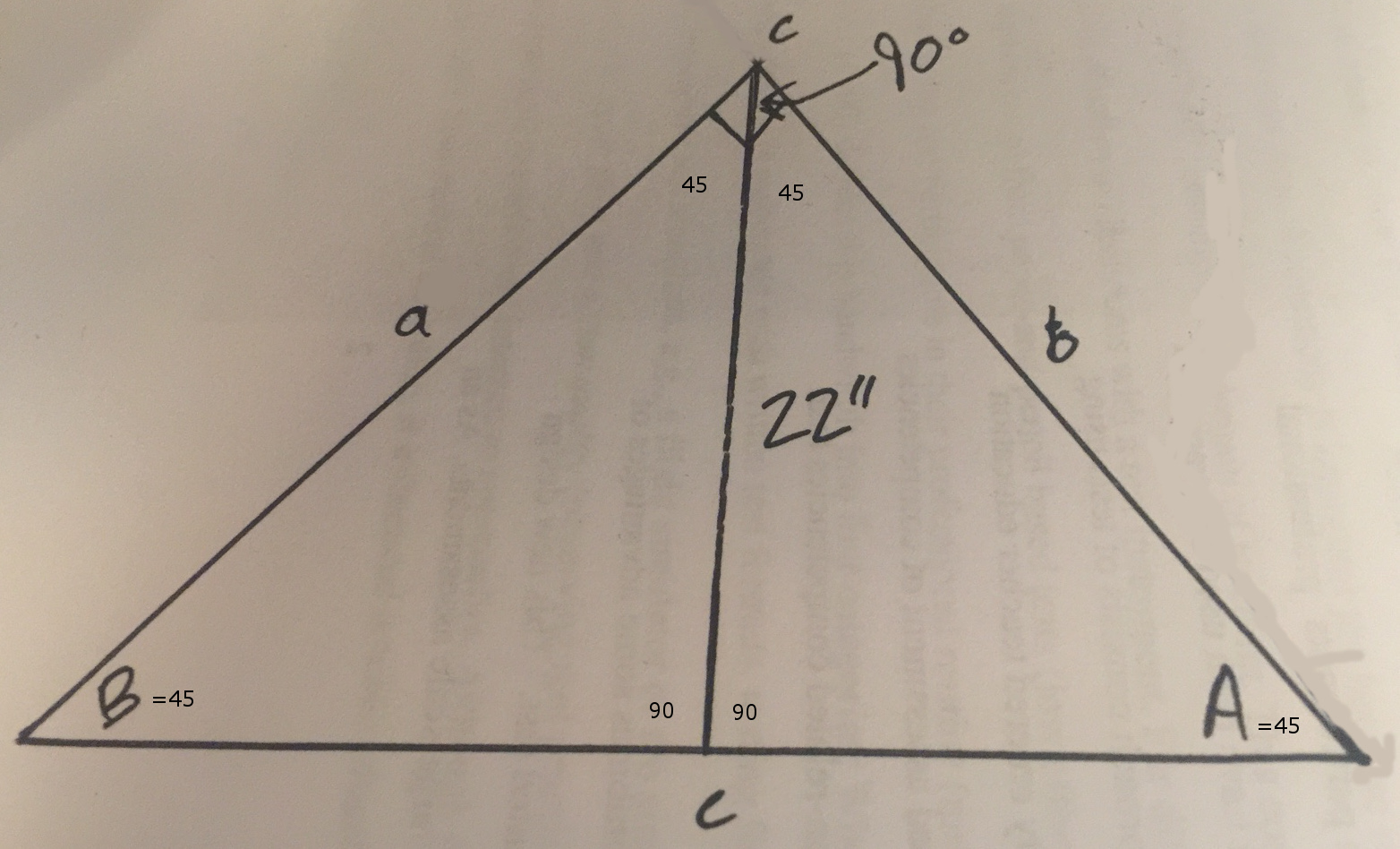
We can divide the larger right-angled triangle into two smaller right-angled triangles, and the 90 degree angle C into two 45 degree angles.

If we take the left side smaller triangle, we know the sizes of two angles and the length of one side. We can use the sine rule to find the length of another side:

`a/(sin 90)=22/(sin 45)`

`a=(22(sin 90))/(sin 45)=(22*1)/0.707=31.1` inches

`b` is the same length as `a`.