How do you find the volume bounded by #y=x^2#, #x=y^2# revolved about the x=-1?

Question

25881 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-07-02T12:57:05

#= 29/30 pi#

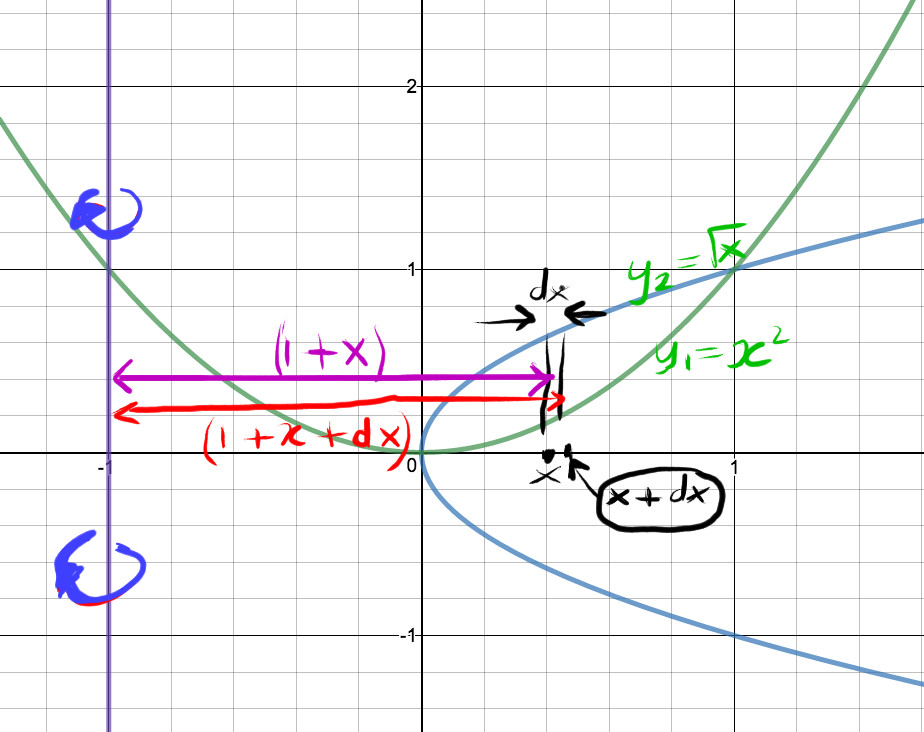
enter image source here

Consider the small element width dx as shown, being revolved around the line x = -1

The cross sectional area (csa) of the washer of width dx is the csa of the outer circle minus the csa of the inner

ie #dA = pi (1 + x + dx)^2 - pi (1 + x)^2#

simplify the algebra with #u = 1+ x# so we have

#dA = pi ((u+dx)^2 - u^2) = pi (2u dx + dx^2)#
#= pi (2 (1+x) dx + dx^2) #

we can already see that #(dA)/dx|_{dx to 0} = pi (2 (1+x) color{red}{+ dx}) #

so we can ignore #mathcal(O)(dx^2)# in the original expression

thus

#dA = 2 pi (1+x) dx #

the volume of that small element is

#dV = (y_2 - y_1) dA#

# = (y_2 - y_1) * 2 pi (1+x) dx #

# = 2 pi (1+x) (sqrt x -x^2) dx #

#\implies V = 2 pi int_0^1 dx qquad (1+x) (sqrt x -x^2) #

#= 2 pi int_0^1 dx qquad (sqrt x -x^2 + x^{3/2} - x^3) #

#= 2 pi (2/3 x^{3/2} -x^3/3 + 2/5x^{5/2} - x^4/4)_0^1 #

#= 2 pi (2/3 - 1/3 +2/5 - 1/4)#

#= 29/30 pi#