Question

How do you find the volume of the region enclosed by the curves `y=2x`, `y=x`, and `y=4` is revolved about the y-axis?

Answer 1

Volume`= 16pi`

Explanation:

The region described is the difference between two inverted cones as can be seen from the diagram below:

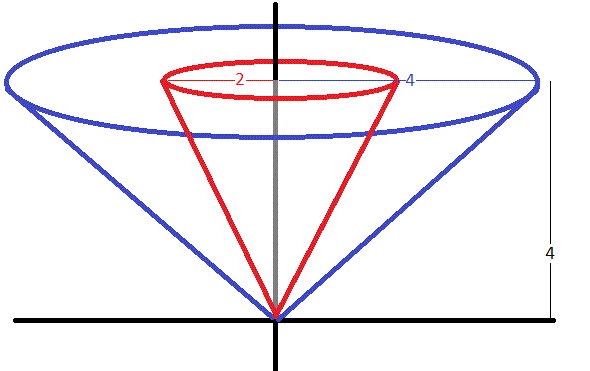
The volume of the bounded region is the difference between the volume of the external cone and the internal cone.

The general formula for the volume of a cone is
`color(white)("XXX")V= (pir^2h)/3`

So the required region has a volume of
`color(white)("XXX")(pi*4^2*4)/3 - (pi*2^2*4)/3`

`color(white)("XXX")=(4pi)/3 * (16-4)`

`color(white)("XXX")=16pi`