Question

How do you find the volume of the solid generated by revolving the region bounded by the curves y = x^(1/2), y = 2, and x = 0 rotated about the x=-1?

Answer 1

`V=(176pi)/15~=36.86`[cubic units]

Explanation:

Refer to the figure below

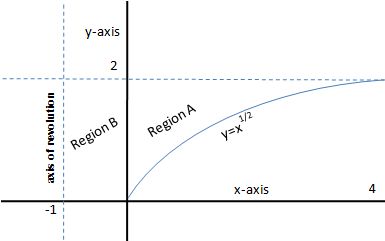
The generator region is Region A limited by `y=x^(1/2)`, `y=2` and `x=0` (the y-axis).

Since the revolution is around an axis parallel to the y-axis, it's convenient to use the inverse function
`f(y)=y^2`

We can use a trick to solve this problem: translating the y-axis to the position of the axis of the revolution. Consequently
`y=(X-1)^(1/2)`
`g(y)=y^2+1`

We only have to remember to exclude Region B, since now the old `x=0` became `x=1`

Applying the formula for volume of a solid of revolution (with a hole), with axis of revolution in y-axis:

`V=pi int_a^b [R(y)^2-r(y)^2]*dy`, where `R(y)` is the external radius and `r(y)` is the internal radius
`V=pi int_0^2 [(y^2+1)^2-1^2]*dy`
`V=pi int_0^2 (y^4+2y^2+cancel1-cancel1)*dy`
`V=pi int_0^2 (y^4+2y^2)*dy`
`V=pi (y^5/5+2/3y^2)|_0^2`
`V=pi(32/5+2/3*8)=pi(96+80)/15=(176pi)/15~=36.86` [cubic units]