Question

How do you find the volume of the solid generated by revolving the region bounded by the graphs `y=e^(x/2), y=0, x=0, x=4`, about the x axis?

Answer 1

Please see below.

Explanation:

Here is a graph of the region in blue. A slice has been taken perpendicular to the axis of rotation. The rotation as shown by the arrow/arc.

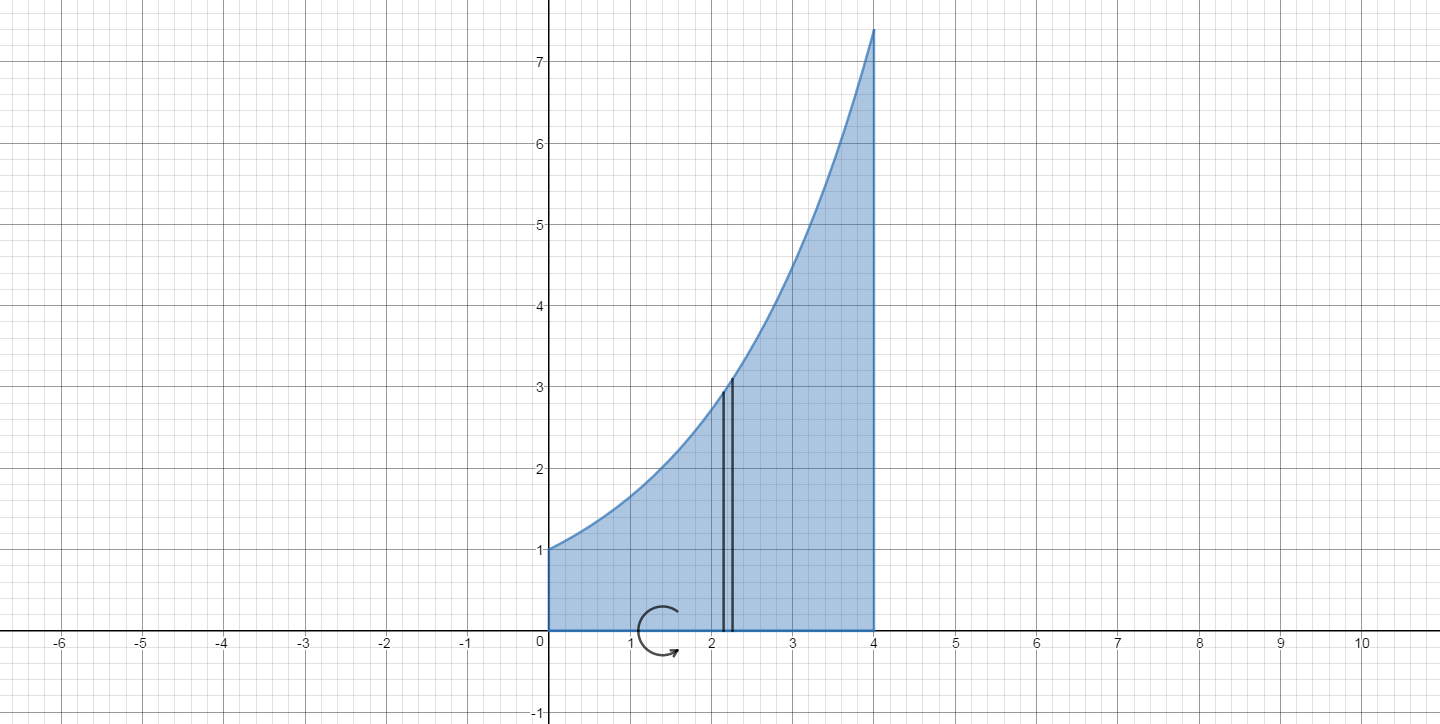
The representative slice is a disc of

thickness dx

and radius

`r =y_"greater" - y_"lesser" = e^(x/2)-0 = e^(x/2)` .

The volume of the representative slice (disc) is

`pir^2"thickness" = pi (e^(x/2))^2 dx = pi e^x dx` .

The values of `x` vary from `0` to `4`, so the resulting solid has volume

`V = int_0^4 pi e^x dx`.

Evaluate the integral to get

`V = pi(e^4-1) ~~ 53.6` (cubic units).