Question

How do you find the volume of the solid in the first octant, which is bounded by the coordinate planes, the cylinder `x^2+y^2=9`, and the plane x+z=9?

Answer 1

The volume is `(81pi)/4 - 9 = 54.6173` (4dp) `unit^3`

Explanation:

The graphs of the plane `x+z=9` and the surface `x^2+y^2=9` are as follows:

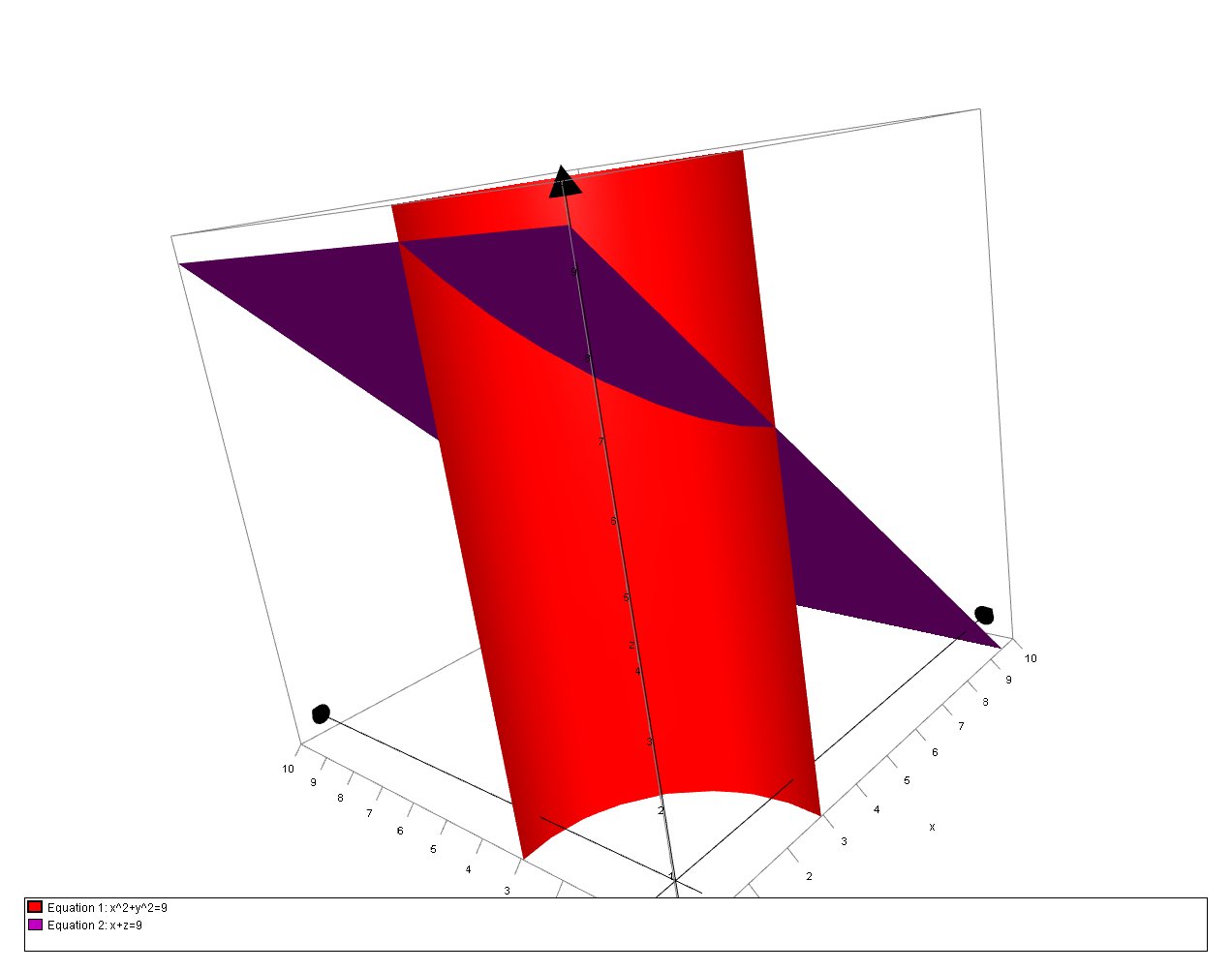
We can us a triple integral to represent the volume as follows:

`v= int int int_R dV`

where

`R={ (x,y,z) | x,y,z>0; x^2+y^2<=9; z<9-x }`

And so we can set up a double integral as follows:

`v= int_a^b int_c^d f(z) \ dx \ dy`
`\ \ = int_a^b int_c^d (9-x) \ dx \ dy`

We now determine the limits of integration by examining a cross section in the `xy`-plane which is a quarter circle of radius 3 centred at the `O`, and so we have:

`0 le x le sqrt(9-y^2)` and `0 le y le 3`

So our integral for the volume is:

`v= int_0^3 int_0^(sqrt(9-y^2)) (9-x) \ dx \ dy`

With nested integral we evaluate from he inside out, so let's deal with the inner integral;

`int_0^(sqrt(9-y^2)) (9-x) \ dx = [9x-1/2x^2]_0^(sqrt(9-y^2))`
`" " = 9(sqrt(9-y^2))-1/2(sqrt(9-y^2))^2`
`" " = 9sqrt(9-y^2)-1/2(9-y^2)`

And so our double integral now becomes:

`v= int_0^3 {9sqrt(9-y^2)-1/2(9-y^2) }\ dy`

And for this integral we can split into the two parts

`I_1 = int_0^3 9sqrt(9-y^2) \ dy` and `I_2 = int_0^3 -1/2(9-y^2) \ dy`

We can just evaluate the second integral to get:

`I_2 = -1/2[ 9y-1/3y^3 ]_0^3`
`\ \ \ = (-1/2){(9)(3)-1/3(27) - 0}`
`\ \ \ = -9`

And for the first integral we use the substitution `y=3sinu`, which gives the result:

`I_1 = 9 int_0^3 sqrt(9-y^2) \ dy`
`\ \ \ = 9 [ysqrt(9-y^2)/2 + 9/2 arcsin(y/3) ]_0^3`
`\ \ \ = 9 {(0+9/2pi/2) - (0+0) }`
`\ \ \ = (81pi)/4`

NOTE - You may also observe that the above integral `int_0^3 sqrt(9-y^2) \ dy` represents the area of a quarter circle of radius `3`, which therefore has area, `A=1/4pi(3^2) = (9pi)/4` which again gives `I_2=9A = (81pi)/4`.

Combining our results gives the total volume as:

`v= (81pi)/4 - 9`
`\ \ = (81pi)/4 - 9`
`\ \ = 54.617251 ...`