Question

How do you find the volume of the solid obtained by rotating the region bounded by the given curves about the specified axis y=x^2, y=1, about y=2?

Answer 1

See below.

Explanation:

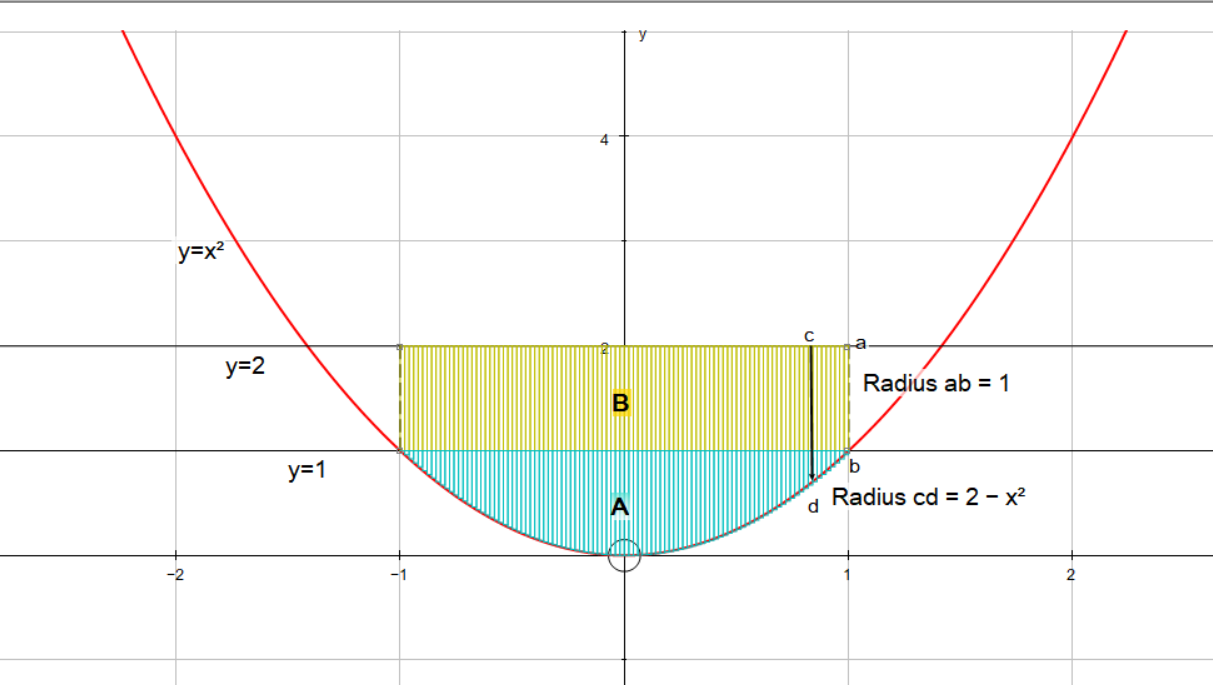
First we find the volume of the area A+B and then subtract the volume of the area B to give us the volume of the area A:

Volume A+B:

It can be see from the diagram that the radius cd of volume A+B is `2-x^2` so the integral will be:

`V=pi*int_(-1)^(1)(2-x^2)^2 dx`

`(2-x^2)^2=4-4x^2+x^4`

`V=pi*int_(-1)^(1)(4-4x^2+x^4) dx=[4x-4/3x^3+1/5x^5]_(-1)^(1)`

`[4x-4/3x^3+1/5x^5]^(1)-[4x-4/3x^3+1/5x^5]_(-1)`

Plugging in upper and lower bounds:

`V=pi*[4(1)-4/3(1)^3+1/5(1)^5]^(1)-[4(-1)-4/3(-1)^3+1/5(-1)^5]_(-1)`

`V=pi*[4-4/3+1/5]^(1)-[-4+4/3-1/5]_(-1)`

`V=pi*[43/15]^(1)-[-43/15]_(-1)=86/15pi`

Volume of B:

This produces a cylinder of radius ab= 1 and length ( this is the length of the interval `[ -1 , 1 ]` which is 2:

`V=pi(1)^2(2)=2pi`

Volume of A= volume(A+B)- volumeB =`(86pi)/15-2pi=color(blue)((56pi)/15)` units cubed.

Volume of A: