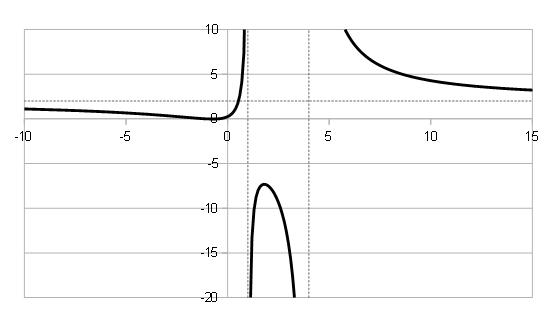
Both the numerator and denominator can be factorized, giving
#((2x+1)(x+1))/((x-1)(x-4))#.
Clearly this has two vertical asymptotes #x=1# and #x=4# (of the simple "#1/x#" type, and two #x#-axis intercepts at #x=1/2# and #x=-1#. Putting #x=0# gives the #y#-axis intercept as #(0,1/4)#,
Since the numerator and denominator have highest powers of #x# which are the same, namely #2#, there is a horizontal asympote #y=2#, the #2# being the ratio of the coefficients of those highest equal powers. (Informally, as #x to oo#, the two squares dominate over the other terms in the expression, so all the #x#s nearly cancel out, leaving the constant #2#.)
Going from left to right, the graph must descend steadily from #y=2# as #x# increases from #-oo#, then go through the two zeroes (and hence through some minimum between the zeroes), thence through the asympototic region at #x=1#, going through a maximum on its way to the second asymptotic region at #x=4#, thence decreasing steadily to the horizontal asymptote as #x to oo#.