Multiply both side by 3 + 4cos(theta):
3r + 4rcos(theta) = 12
Substitute x for rcos(theta):
3r + 4x = 12
Subtract 4x from both sides:
3r = 12 - 4x
Square both sides:
9r^2 = 144 - 96x + 16x^2
Substitute 9x^2 + 9y^2 for 9r^2:
9x^2 + 9y^2 = 144 - 96x + 16x^2
Move everything but the constant to the left and subtract 7h^2 from both sides:
-7x^2 + 96x - 7h^2 + 9(y - 0)^2 = 144 - 7h^2
Complete the square for the x term:
-7(x^2 - 96/7x + h^2) + 9(y - 0)^2 = 144 - 7h^2
-2hx = -96/7x
h = 48/7
-7(x - 48/7)^2 + 9(y - 0)^2 = -1296/7
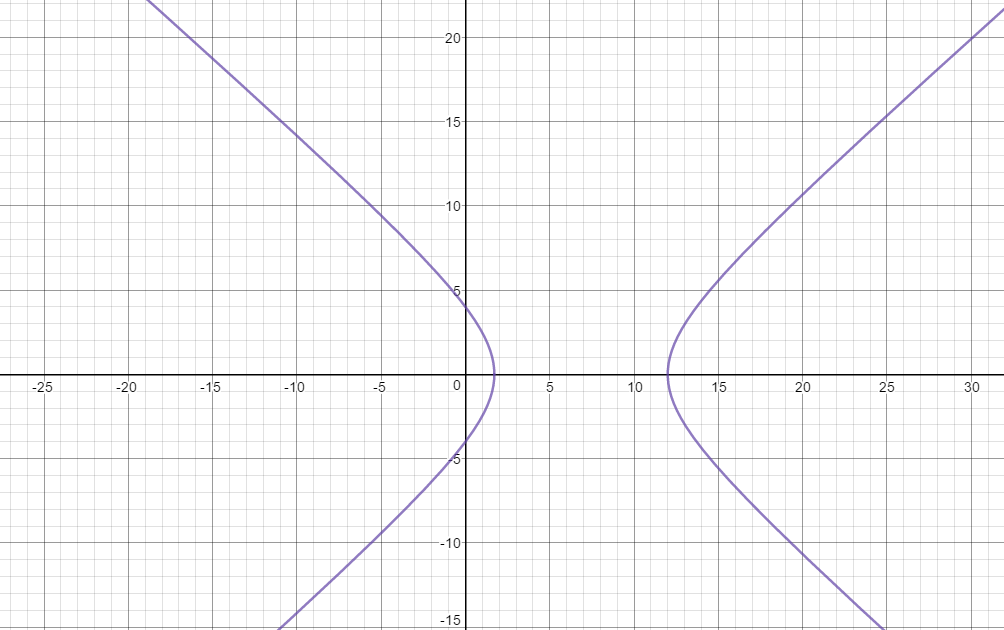
(x - 48/7)^2/(36/7)^2 - (y - 0)^2/(12sqrt(7)/7)^2 = 1
Center(48/7, 0)
x_1 = 48/7 - 36/7 = 12/7
x_2 = 48/7 + 36/7 = 12
Vertices: (12/7, 0) and (12,0)
b/a = (12sqrt(7)/7)/(36/7) = sqrt(7)/3
asymptotes:
y = -(sqrt(7)/3)(x - 48/7)
y = (sqrt(7)/3)(x - 48/7)
Here is the graph:
 )
)
 )
) 