Question

How do you graph `(x^2-4)/(x^2-9)`?

Answer 1

You find the intercepts and the asymptotes, and then you sketch the graph.

Explanation:

Step 1. Find the `y`-intercepts.

`y = f(x) = (x^2-4)/(x^2-9)`

`f(0) = (0^2-4)/(0^2-9) = (-4)/-9 = 4/9`

The `y`-intercept is at (`0,4/9`).

Step 2. Find the x-intercepts.

`0 = (x^2-4)/(x^2-9)`

`x^2-4 = 0`

`x^2 = 4`

`x = ±2`

The `x`-intercepts are at (`-2,0`) and (`2,0`).

Step 3. Find the vertical asymptotes.

Set the denominator equal to zero and solve for `x`.

`x^2 -9 = 0`

`x^2 = 9`

`x = ±3`

There are vertical asymptotes at `x = -3` and `x = 3`.

Step 4. Find the horizontal asymptote.

Both equations are of the second order, so we divide the coefficients of the `x^2` terms.

`1/1 = 1`

The horizontal asymptote is at `y=1`.

Step 5. Draw your axes and the asymptotes.

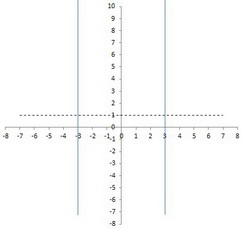
The vertical asymptotes divide the graph into three regions of `x`s.

Step 6. Sketch the graph in the each region.

(a) In the left hand region,

`f(-4) = (16-4)/(16-9) = 12/7 ≈ 1.7`.

The point at (`-4,1.7`) is in the second quadrant, so we have a "hyperbola" above the horizontal asymptote.

(b) In the right hand region,

`f(4) = (16-4)/(16-9) = 12/7 ≈ 1.7`.

So we have a mirror-image "hyperbola" in the first quadrant.

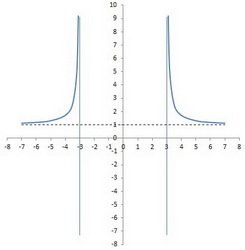
(c) In the middle region, we have

`f(0) = 4/9 ≈ 0.44` and

`f(-1) = f(1) = (1-4)/(1-9) =(-3)/-8 ≈ 0.38`

The points at (`-1,0.38`) and (`1,0.38`) are below the `y`-intercept, so we have an "inverted parabola" between the vertical asymptotes.

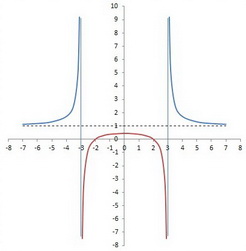
And we have our graph.