How do you simplify #sqrt(108x^3y^5 )#?

Question

2776 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-01-28T12:36:05

#6xy^2sqrt(3y)#

We are looking for values that are squared. These can be taken outside the square root in non-squared form.

Using a factor tree to deal with the number 108
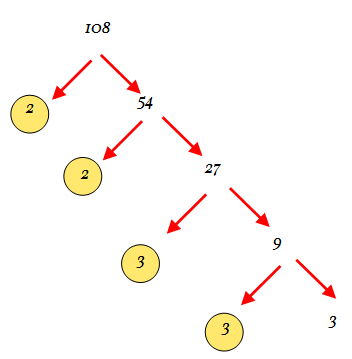
Tony B

So the prime factors are: #2^2xx3^2xx3#

#x^3# is the same as: #x^2xx x#
#y^5# is the same as: #y^2xxy^2xxy#

Thus write: #sqrt(108x^3y^5)# as #sqrt(2^2xx3^3xx3xx x^2xx x xxy^2xxy^2xxy)#

Taking the squared values outside the root gives:

#2xx3xx x xxy^2xxsqrt(3xy)#

#6xy^2sqrt(3xy)#