How do you sketch the angle whose terminal side in standard position passes through #(2,-sqrt5)# and how do you find sin and cos?

Question

2252 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-08-31T15:09:52

Letting the angle be #alpha#, we get:
#sinalpha = -sqrt(5)/3#
#cosalpha = 2/3#

Draw a diagram:

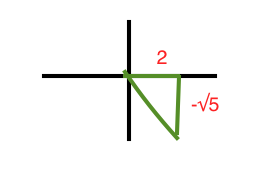
enter image source here

Since the angle between the two known sides is right, we can use pythagorean theorem to determine the length of the hypotenuse.

Let the hypotenuse be #x#.

#(2)^2 + (-sqrt(5))^2 = x^2#

#4 + 5 = x^2#

#x^2 = 9#

#x = +-3#

A negative hypotenuse is impossible, so #x = 3#, or the hypotenuse has a measure of #3# units.

Note that the angle in standard position would be the angle of the triangle opposite the #-sqrt(5)#.

Let the angle be #alpha#.

#sinalpha = -sqrt(5)/3#

#cosalpha = 2/3#

Hopefully this helps!