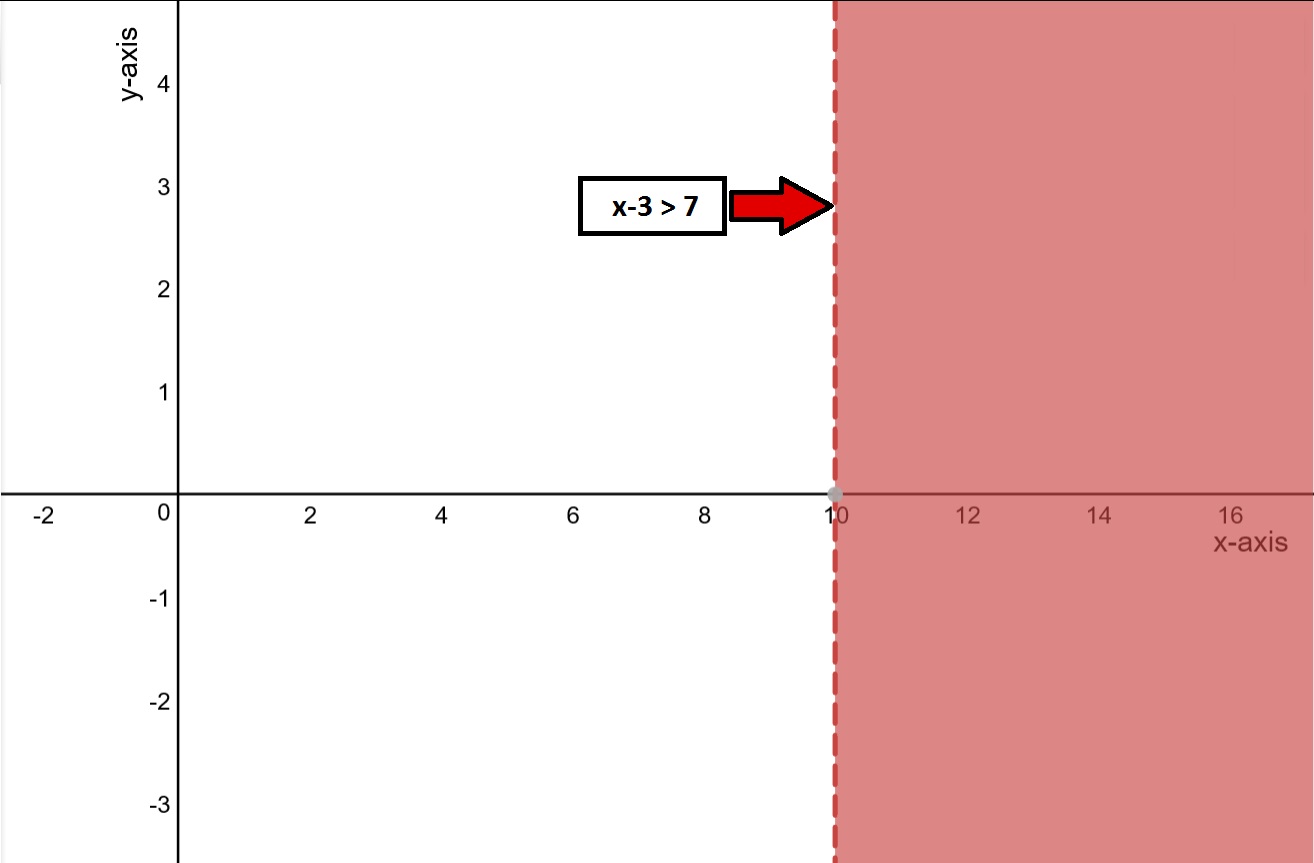
How do you solve and graph #x-3>7#?
1 Answer
Express the inequality as an equation, then test points to determine where to shade the graph.
Explanation:
Strategy: Express the inequality as an equation (i.e., with an equals sign). Graph the equation. Strict inequality lines are drawn as a dashed line. Shade the side of the line where the inequality is true.
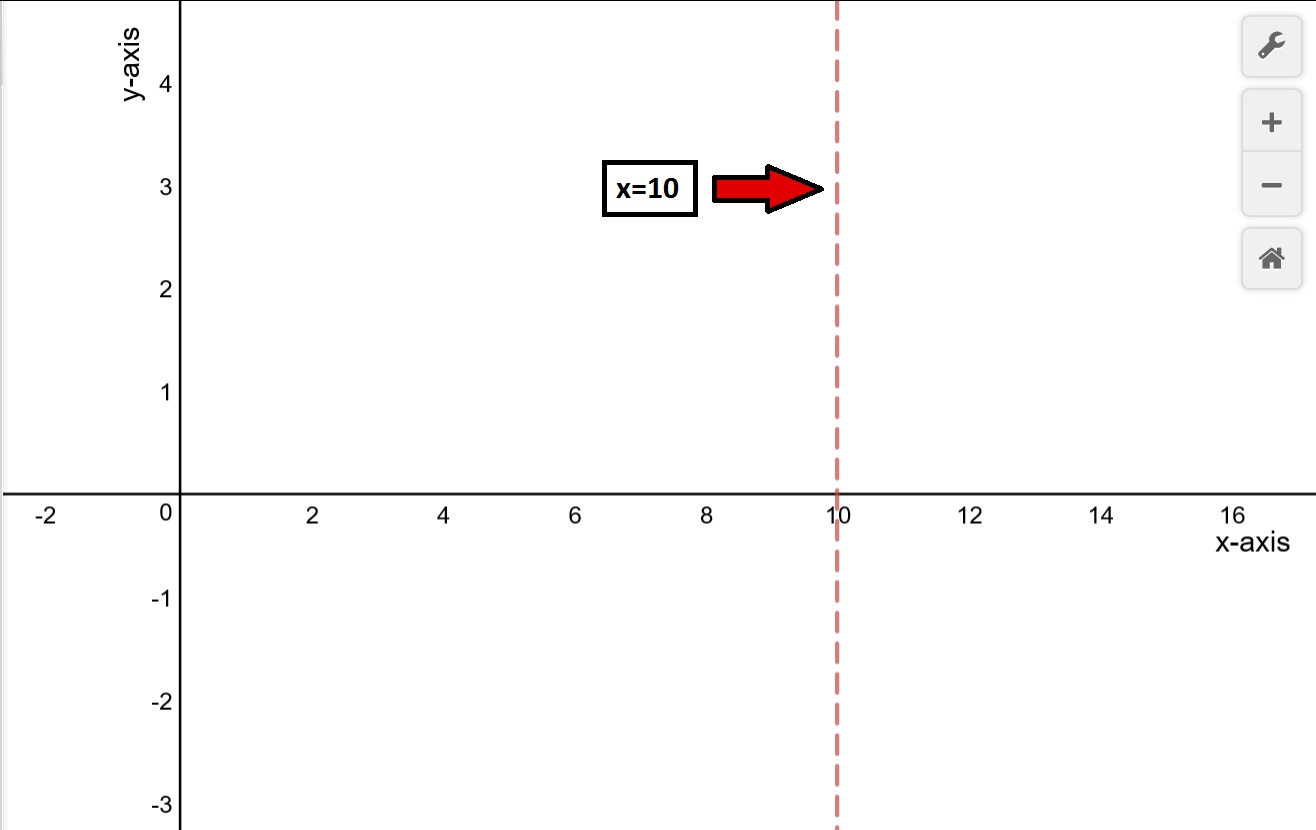
Step 1. Express the inequality as an equation.
Step 2. Graph the equation. Because
Step 3. Shade the side of the line where the inequality is true.
Trying
So don't shade that side of the line. However, trying the point