How do you solve #(p-5)/(3-p)<=0# using a sign chart?
1 Answer
Have a line in the chart for each the numerator and denominator separately, then one for their quotient. The result is between p=3 and p=5, also including p=5.
Explanation:
Since we can tell the sign of a quotient based on the signs of the numerator and denominator we can use this to create the sign chart as shown:
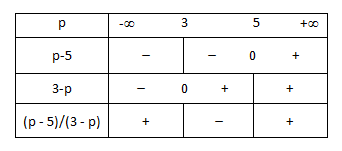
From the singn chart you can see that the graph is below zero (or negative) between p=3 and p=5.
Additionally, we have to investigate the roots to see when the graph is equal to 0, since the question was
So at p=3 the denominator is 0 while the numerator is not, resulting in an indefinite solution. (This would appear as an asymptote approaching negative infinity in this case).
At p=5 the numerator is 0, meaning the graph will be equal to 0 as well.
The overall result is the graph is

