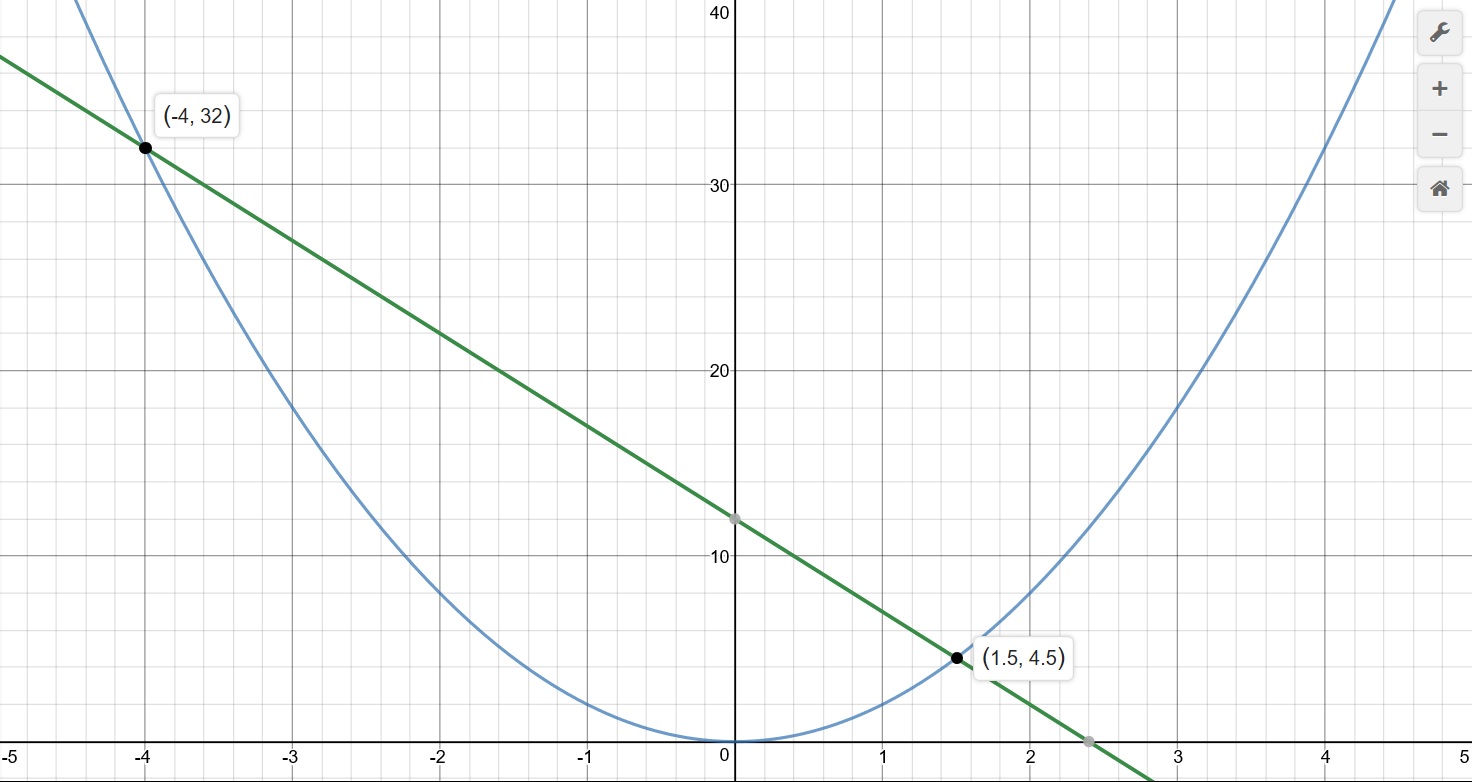
How do you solve the equation #2x^2=-5x+12# by graphing?
1 Answer
Nov 13, 2017
The intersection points occur at
Explanation:
There are several ways to graph this equation. One way that is popular is to graph the left hand side separately from the right and observe where they intersect with each other.
Graphing
graph{y=2x^2[-5,5,-5,40]}
Graphing
graph{(y-2x^2)(y+5x-12)=0[-5,5,-5,40]}
The intersection points occur at
One very easy way to see these is to plot both equations in Desmos.com, which gives the following interactive graph.