Question

How do you solve the inequality: `(x - 1) (x + 4) < 0`?

Answer 1

`-4 < x < 1`

Explanation:

Note that the left side of this inequality would be equal to `0`
if `x=-4` or `x=1`

We could divide the number line up into 3 ranges :

#{: ("Range",color(white)("XXX"),(x-1), color(white)("XXX"),(x+4),color(white)("XXX"),"product"), ("A",color(white)("XXX"),< 0, color(white)("XXX"),< 0,color(white)("XXX"),<0), ("B",color(white)("XXX"),< 0,color(white)("XXX"),> 0,color(white)("XXX"),< 0), ("C",color(white)("XXX"),> 0, color(white)("XXX"),> 0, color(white)("XXX"),> 0) :}#

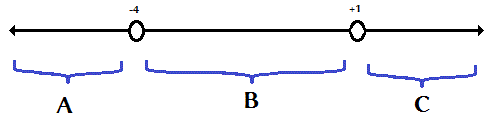
Only in Range "B" is the product `(x-1)(x+4) < 0`