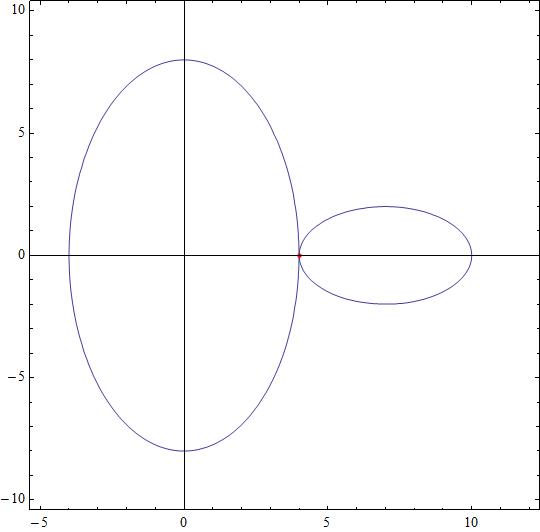
How do you solve the system #4x^2-56x+9y^2+160=0# and #4x^2+y^2-64=0#?
2 Answers
Case 1:
Case 2:
The only real solution is
Thus, the solution set is
Hopefully this helps!
Explanation:
Making
we obtain
Now we solve
obtaining
and
or
we have
Case 1:
Case 2:
The only real solution is
Thus, the solution set is
Hopefully this helps!
Making
we obtain
Now we solve
obtaining
and
or
we have
