How do you solve the system of linear equations #x+y=-8# and #4x+4y=-8#?
2 Answers
I don't believe this system of equations can be solved.
Explanation:
We could normally multiply one equation by a constant to eliminate one variable by subtracting the result from the other equation, but any time we try that with this system of equations we eliminate both variables.
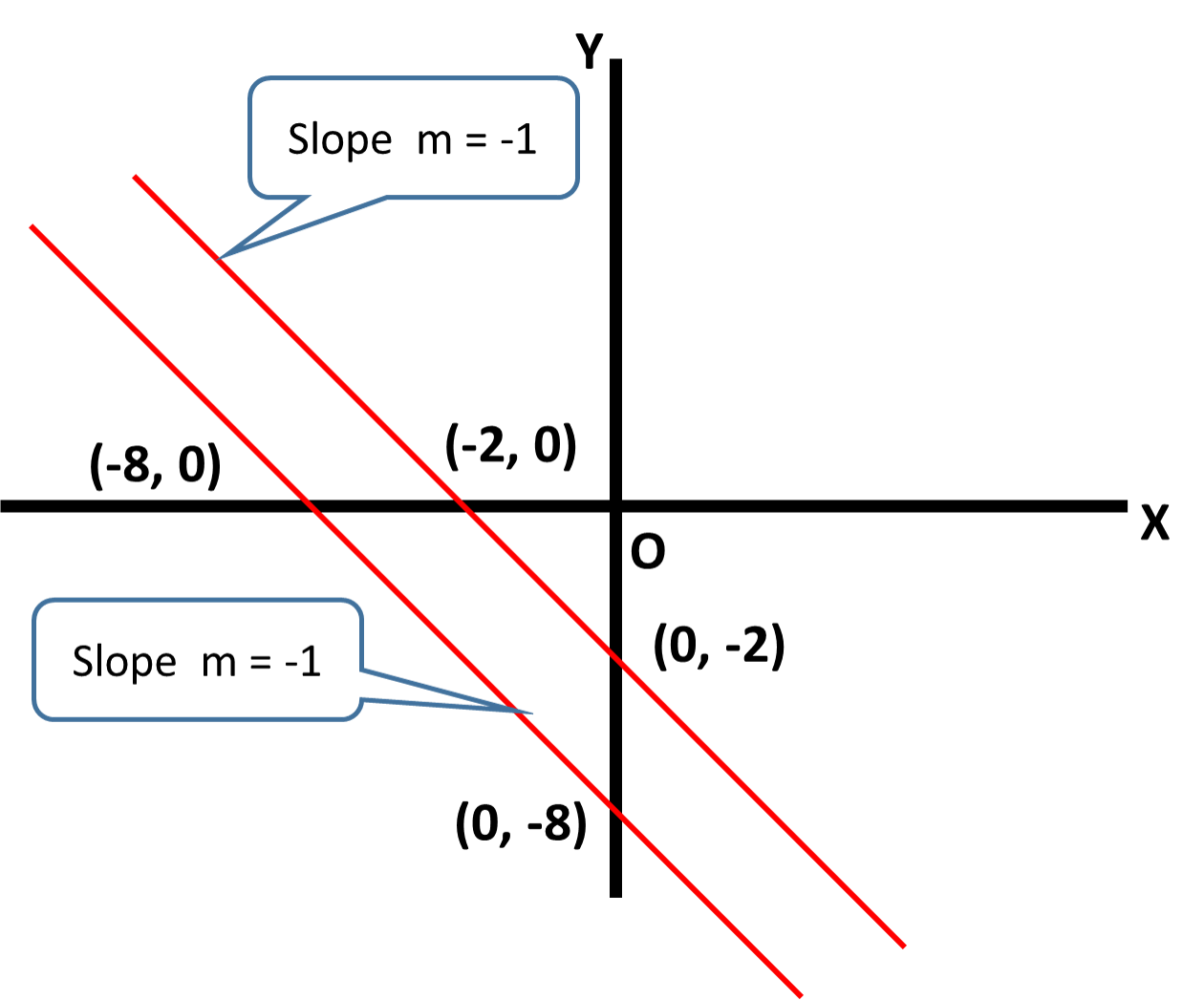
Here's another way of thinking about it: these are the equations of lines, and both lines have the same slope (gradient), that is, they are parallel lines, so they never cross, and when solving systems of linear equations, what we are finding is the point where the lines cross.
Let's just make that a bit more explicit by rearranging the equations into point-slope form for lines:
First equation:
Second equation:
These are the equations of two lines both with slope =
No solution. The two lines are parallel.
Explanation:
Given -
#x+y=-8# ------------------ (1)
#4x+4y=-8# --------------(2)
The slope of the Lines is