Question

How do you solve `(x+5)^2 /( x^2-4 )>=0`?

Answer 1

Solve `F(x) = (x + 5)^2/(x^2 - 4) >= 0`

Ans: Open intervals: (-inf, -2) and (2, +inf)

Explanation:

`F(x) = (x + 5)^2/((x - 2)(x + 2)) >= 0`.
This function is undefined when `x = +- 2`
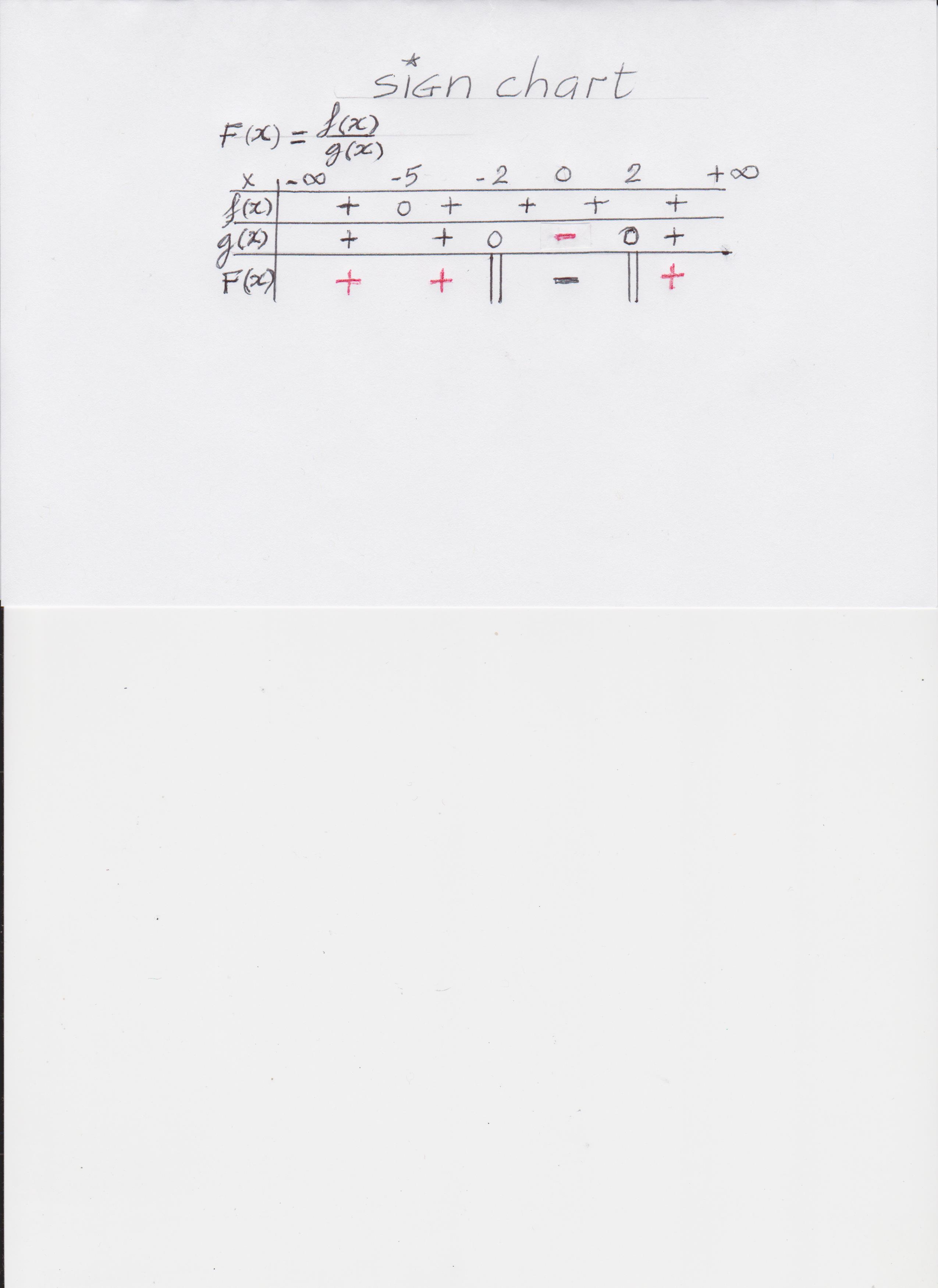
Solve this inequality algebraically by using a sign chart.
Call `f(x) = (x + 5)^2` . This function is always positive
Call `g(x) = (x - 2)(x + 2)`. This g(x) = 0 when `x = +-2`
g(x) < 0 between (-2) and (2).
Sign Chart. of `F(x) = f(x)/(g(x))`
Sign of F(x) is the resultant sign of f(x) and g(x).
F(x) is positive in interval (-inf, -2) and F(x) > 0 inside (2, +inf).
Ans: Open Intervals: (-inf, -2) and (2, +inf).