Question

How do you use the distributive property to simplify `5(6-3i)+3(i-8)`?

Answer 1

`6 - 12i`

Explanation:

I'm not sure if your `i` is just a variable or if it is an imaginary number, but either way that will not affect the simplified answer.

To simplify this, use the distributive property (shown below):

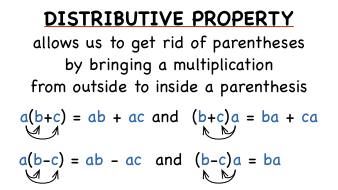
Following this image, we know that:
`color(blue)(5(6-3i) = (5 * 6) + (5 * -3i) = 30 - 15i)`
and
`color(blue)(3(i-8) = (3 * i) + (3 * -8) = 3i - 24)`

Now combine them:
`30 - 15i + 3i - 24`

Color-code the like terms:
`color(red)(30) quadcolor(green)(-quad15i) quadcolor(green)(+quad3i) quadcolor(red)(-quad24)`

Combine the like terms:
`6 - 12i`

Hope this helps!