How do you use the method of cylindrical shells to find the volume of the solid obtained by rotating the region bounded by #y =1/(x^2+1)#, x=0, x=1, y=0 revolved about the y-axis?
1 Answer
Volume
Explanation:
If you imagine an almost infinitesimally thin vertical line of thickness
#delta A ~~"width" xx "height" = ydeltax = f(x)deltax#
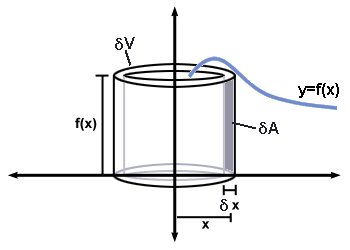
If we then rotated this infinitesimally thin vertical line about
#delta V~~ 2pi xx "radius" xx "thickness" = 2pixdeltaA=2pixf(x)deltax#
If we add up all these infinitesimally thin cylinders then we would get the precise total volume
# V=int_(x=a)^(x=b)2pixf(x) dx #
So for this problem we have:
# V = int_0^1 2pix 1/(x^2+1) dx #
# \ \ \ = pi int_0^1 (2x)/(x^2+1) dx #
# \ \ \ = pi ln|x^2+1)|_0^1 #
# \ \ \ = pi (ln2-ln1)#
# \ \ \ = piln2#

