Question

How do you write a nuclear equation for the alpha decay of `"_62^148Sm`?

Answer 1

`""_ (color(white)(1)62)^148"Sm" -> ""_ (color(white)(1)60)^144"Nd" + ""_2^4alpha`

Explanation:

The thing to remember about alpha decay is that it occurs when the nucleus of a radioactive nuclide emits an alpha particle, `alpha`, which is essentially the nucleus of a helium-4 atom.

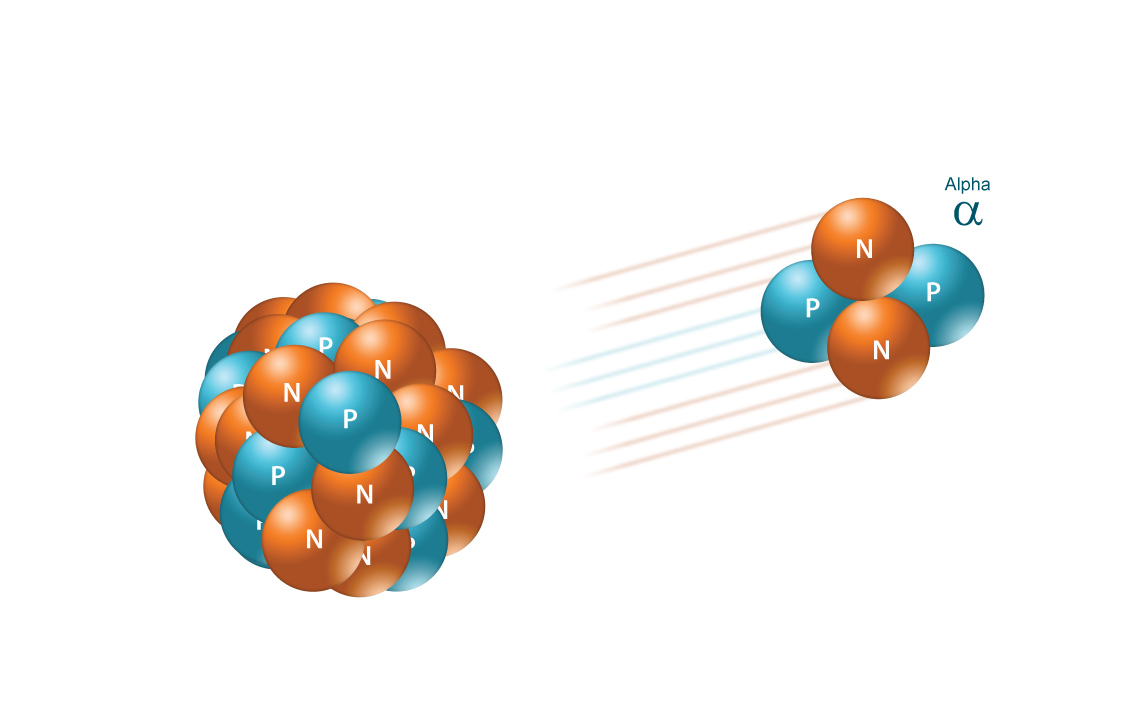
Simply put, an alpha particle contains `2` protons and `2` neutrons, which implies that it has a mass number equal to `4`.

Therefore, you can use isotopic notation to write the alpha particle using its atomic number of `2` and mass number of `4`

`""_2^4alpha`

You can now set up the nuclear equation that describes the alpha decay of samarium-148

`""_ (color(white)(1)color(blue)(62))^color(orange)(148)"Sm" -> ""_color(blue)(Z)^color(orange)(A)"X" + ""_color(blue)(2)^color(orange)(4)alpha`

In order to find the identity of the daughter nuclide, use the fact that mass and charge are conserved in a nuclear equation

`color(orange)(148 = A + 4)" " ->` conservation of mass

`color(white)(1)color(blue)(62 = Z + 2)" " ->` conservation of charge

Solve to find the values of `A` and `Z`

`148 = A + 4 implies A = 144`

`color(white)(1)62 = Z + 2 implies Z = 60`

Grab a periodic table and look for the element which has the atomic number equal to `60`. This element is neodymium, `"Nd"`. The daughter nuclide is neodybium-144.

The balanced nuclear equation that describes the alpha decay of samarium-148 will thus be

`color(darkgreen)(ul(color(black)(""_ (color(white)(1)62)^148"Sm" -> ""_ (color(white)(1)60)^144"Nd" + ""_2^4alpha)))`