Question

How do you write the solution in interval notation, and graph `-5/3x<=-10`?

Answer 1

Interval notation: `[6,∞)`

See the graph below.

Explanation:

First, solve for x:

`-5/3x<=-10`

When dividing or multiplying by a negative, then flip the inequality sign.

`(-5/3color(red)(*-3/5))x<=-10(color(red)(-3/5))`

`x>=6`

To write interval notation, use brackets `[]` and parenthesis `()`. Brackets are used when the answer is included, and parenthesis are used when the answer is excluded. Interval notation goes from least to greatest.

In this case, the answer is included. The answer also goes up to infinity, which will always have a parenthesis, as you cannot reach infinity:

`[6,∞)`

This means that any number from `6` to `∞` is an answer, including `6` and excluding `∞`.

A graph would look like this:

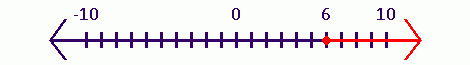
The dot at 6 is shaded in as 6 is included in the answer.