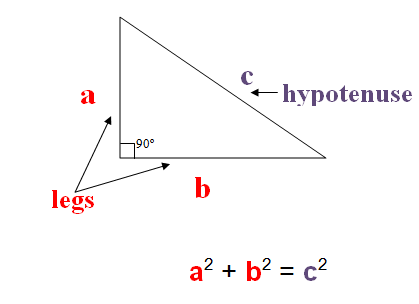
How does the length of the hypotenuse relate to the length of the legs?

Question

How does the length of the hypotenuse relate to the length of the legs?

1 Answer

Impact of this question

7182 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-01-03T07:41:57

The relation is called the Pythagorean Theorem. In any right triangle, the following relation is true:

$a^{2} + b^{2} = c^{2}$ $a^2+b^2=c^2$

where $a$ $a$ and $b$ $b$ are the legs (shorter sides) of a right triangle and $c$ $c$ is the hypotenuse (the longest side, which is opposite the right angle).

For example, a triangle with sides $3, 4$ $3,4$ and $5$ $5$ can be shown to be a right triangle.

The legs of the triangle are $3$ $3$ and $4$ $4$ , so $a = 3$ $a=3$ and $b = 4$ $b=4$ . The longest side of the triangle, $5$ $5$ , is the hypotenuse, so $c = 5$ $c=5$ .

$a^{2} + b^{2} = c^{2}$ $a^2+b^2=c^2$
$3^{2} + 4^{2} = 5^{2}$ $3^2+4^2=5^2$
$9 + 16 = 25$ $9+16=25$
$25 = 25$ $25=25$

lrd.kangan.edu.au

historyofpythagoreantheorem.weebly.com

Science

Math

Humanities

... and beyond

How does the length of the hypotenuse relate to the length of the legs?

1 Answer

Related questions

Impact of this question