Question

How length of /AH/ = ?

Answer 1

E) `8sqrt2` cm

Explanation:

For an isosceles triangle, the area will be maximum when it is a right triangle, `=> angleBAC=90^@`
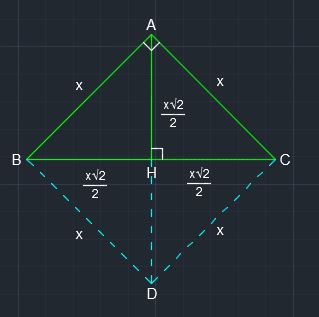
As shown in the figure, `ABCD` is a square, `AD and BC` are the diagonals,
Let `AB=AC=x, => AD=BC=sqrt2x`,
`=> AH=(AD)/2=(sqrt2x)/2`,
given maximum area of `DeltaABC=128`,
`=> 1/2*BC*AH=128`
`=> 1/2*sqrt2x*(sqrt2x)/2=128`
`=> 1/2*x^2=128`
`=> x=16` cm
`=> AH=(sqrt2x)/2=(sqrt2*16)/2=8sqrt2` cm

Sol. 2)

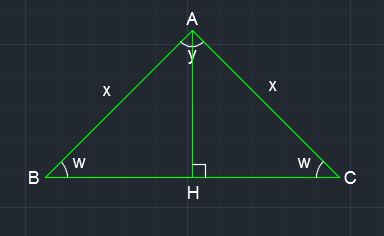
Let `AB=AC=x`,
`=>` area `DeltaABC=1/2*x^2*siny`
as maximum value of `siny` is 1, when `siny=1`, maximum area of `DeltaABC` can be obtained.
`=> siny=1, => y=90^@`
`=> 1/2*x^2=128, => x=sqrt256=16`
`=> angleABC=angleACB=90/2=45^@`
`=> AH=xsinw=16sin45=16*sqrt2/2=8sqrt2` cm