There shouldn't be, and that's why "B"_2 is very unstable; it has two orthogonally-localized half-pi bonds, which is quite a weak bond.
Imagine that each "B" takes the place of a "CH" on "HC"-="CH".
 )
)
Then, take away the sigma bond, the two "H" atoms, and one electron from each of the two full pi bonds, and you have a structure similar to "B"_2.
What about "Li"_2 "molecule"? Is that stable? (Its bond length is "267.3 pm", over twice the length of an average bond.)
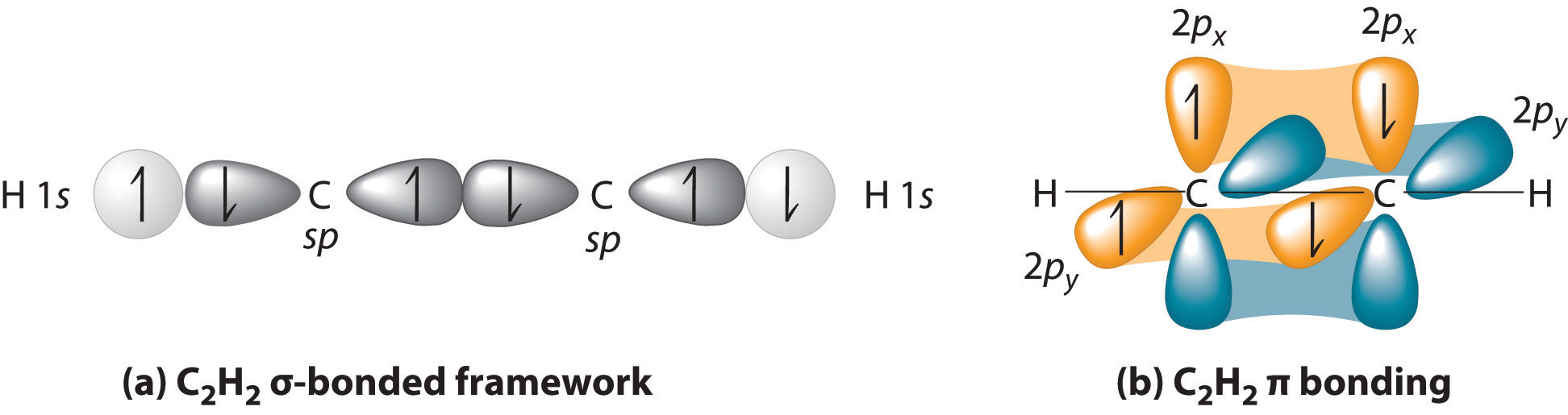
The first chemical bond made in a molecule is preferentially a bbsigma bond.
sigma bonds are formed from a direct atomic orbital overlap. In comparison, pi bonds are sidelong overlaps and thus, sigma overlaps are made preferentially because they form the stronger bond.
![]()
"B"_2 contains two boron atoms, which each use a basis of a 1s, a 2s, and three 2p atomic orbitals.
For "Li"_2 through "N"_2, there exists an orbital mixing effect that makes the sigma molecular orbital for a 2p_z-2p_z overlap (sigma_(g(2p))) higher in energy than the pi molecular orbitals for a 2p_(x//y)-2p_(x//y) overlaps (pi_(u(2p))).
![Inorganic Chemistry, Miessler et al., Ch. 5.2.3]()
So, this particular orbital energy ordering takes place in the molecular orbital diagram, similar to the "C"_2 molecule:
![]()
where |E_(sigma_(2p_z))| > {|E_(pi_(2p_x))| = |E_(pi_(2p_y))|}.
Since "C" has one more electron, "B"_2 would have a similar MO diagram, EXCEPT for two fewer electrons. This, at first glance, seems to suggest that "B"_2 has two half-pi bonds, with a molecular electron configuration of:
color(green)((sigma_(1s))^2(sigma_(1s^"*"))^2(sigma_(2s))^2(sigma_(2s^"*"))^2(pi_(2p_x))^1(pi_(2p_y))^1)
Since "B"_2 is paramagnetic with two electrons, in order to make that bond, there are indeed two half-bbpi bonds, which form what we represent improperly in line notation as a sigma bond... and it isn't actually a sigma bond.
:"B"-"B":
That isn't a sigma bond, but two half-pi bonds. We would then expect "B"_2 to be very unstable, which it is. (The lone pairs are from the sigma_(2s) and sigma_(2s)^"*", since bonding + antibonding filled = nonbonding.)
 )
)

