Question

How to determine the heat evolved or consumed by the reaction of 1.0 g SO2(g) with excess oxygen, with results from a Hess' Law Equation?

Answer 1

(A)

Based off of the state function property of enthalpy, Hess's Law states that you can:

• Scale a reaction stoichiometry `->` scale the enthalpy value
• Reverse a reaction `->` Reverse the sign of the enthalpy

And if you follow these two operations, you preserve the validity of your answer.

The reactions you have available are:

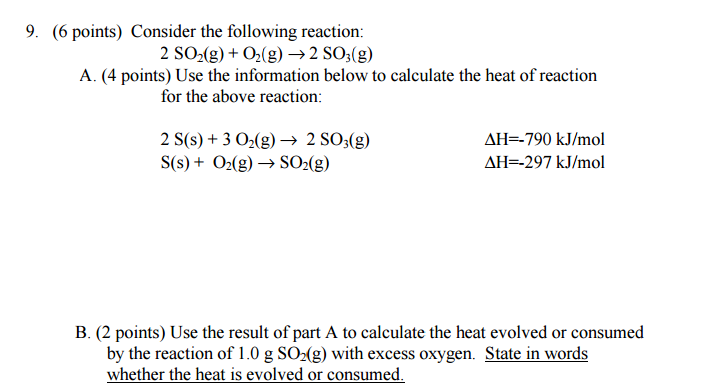
(1) `2"S"(s) + 3"O"_2(g) -> 2"SO"_3(g)`, `Delta"H"_1 = -"790 kJ/mol"`
(2) `"S"(s) + "O"_2(g) -> "SO"_2(g)`, `Delta"H"_2 = -"297 kJ/mol"`

to achieve:

(3) `2"SO"_2(g) + "O"_2(g) -> 2"SO"_3(g)`, `Delta"H"_"rxn" = ???`

These reactions are indeed error-free to begin with, so let's proceed.

• Notice again, that the sulfur solid is NOT in the final result. Thus, it must have disappeared in the middle of the reaction as an intermediate. So... you must have to cancel that out.
• In (2), `"SO"_2(g)` is a product, whereas in (3), it's a reactant. Thus, you must reverse (2).
• To cancel out `"S"(s)` now, you should double the coefficients in (2).

Thus, your final operations become:

`cancel(2"S"(s)) + 3"O"_2(g) -> 2"SO"_3(g)`
`2("SO"_2(g) -> cancel("S"(s)) + "O"_2(g))`
`"--------------------------------------"`
`color(blue)(2"SO"_2(g) + "O"_2(g) -> 2"SO"_3(g))`

Convince yourself that the sulfur and oxygen are balanced!

Now, you can proceed to calculate the enthalpy:

`color(blue)(Delta"H"_"rxn") = Delta"H"_1 + (-2)Delta"H"_2`

`= -790 + (-2)(-297) "kJ/mol"`

`=` `color(blue)(-"196 kJ/mol")`

Also, be sure to clarify whether it's a standard reaction (i.e. the pressure according to STP, and `25^@ "C"`) or not. I think you were missing that, because there is no specified temperature in this question.

(B)

In this part, in knowing that you use "excess oxygen", you assume that `"SO"_2(g)` is the limiting reagent (i.e. it is entirely consumed first, and the reaction ends after that point), and from there, utilize the following equation for heat flow at a constant pressure:

`\mathbf(Delta"H"_"rxn" = (q_"rxn")/"mols limiting reagent" = (q_"rxn")/(n_("SO"_2(g))))`

I assume the reaction conditions (i.e. temperature and pressure) of part (A) and (B) are the same.

Thus, we should get for the heat flow involved in this reaction:

`color(blue)(q_"rxn") = n_("SO"_2(g))Delta"H"_"rxn"`

`= stackrel(n_("SO"_2(g)))overbrace((1.0 cancel("g SO"_2))((cancel("1 mol SO"_2))/(64.063 cancel("g SO"_2))))stackrel(Delta"H"_"rxn")overbrace((-"196 kJ/"cancel"mol"))`

`= color(blue)(-"3.06 kJ")`

Since the sign of the heat flow is negative, heat is produced by the reaction and leaves the reaction. You can decide what the wording is for your answer.