How to find b in linear equation form y=mx+b if the 2 coordinates are (5,6) and (1,0)?

1 Answer
sankarankalyanam
Apr 14, 2018

color(blue)(y = (3/2)x - (3/2)

color(purple)( " is the slope-intercept form of equation with slope = 3/2, y-intercept = -3/2"

Explanation:

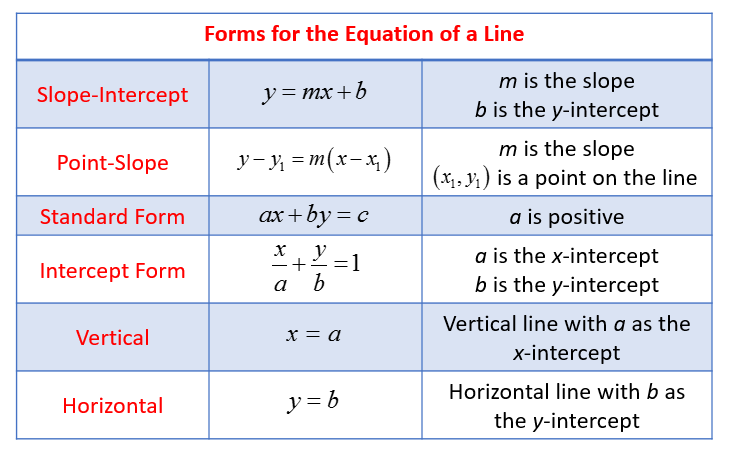
![https://www.onlinemathlearning.com/http://equation-of-a-line-types.html](https://useruploads.socratic.org/Lom1sqzqSY2elGYpMcoQ_forms-equation-of-line.png)

(x_1,y_1) = (5,6), (x_2,y_2) = (1,0)

Equation of line is (y-y_1) / (y_2 - y_1) = (x-x_1) / (x_2-x_1)

(y - 6) / (0-6) = (x-5) / (1-5)

(y-6) /cancel( -6 )^color(red)(3)= (x-5) /cancel( -4)^color(red)(2)

2y - 12 = 3x - 15, " cross multiplying"

2y = 3x - 15 + 12

Standard form of slope-intercept equation is color(indigo)(y = mx + c
color(blue)(y = (3/2)x - (3/2)

color(purple)( " is the slope-intercept form of equation with slope = 3/2, y-intercept = -3/2"

Related questions
See all questions in Slope-Intercept Form
Impact of this question
19059 views around the world
You can reuse this answer
Creative Commons License