#-2x^2+8x -6:#
factorise:
#-2(x^2-4x+3)#
#(-4)/2 = -2#
#x^2-4x + 4 = (x-2)^2#
#x^2 - 4x + 3 = (x-2)^2 - 1#
#-2(x^2-4x+3) = -2((x-2)^2-1)#
#=-2(x-2)^2 + 2#
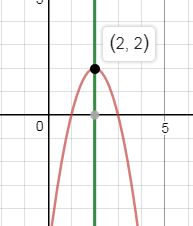
#a(x-h)^2 + k = -2(x-2)^2 + 2#
turning point: #(-h,k)#, where #x=h# is the axis of symmetry.
#(-h, k) = (2,2)#
#x= 2# is the axis of symmetry.
since the coefficient of #x^2# is negative #(-2)#, the graph opens to the bottom.
the point #(-h, k)# is therefore a maximum point.
since the maximum point is the highest possible, the range is equal to or below #2#.
#{y: y<=2}#
#6x^2+3x-18:#
factorised:
#6(x^2+0.5x-3)#
#x^2+0.5x+0.0625 = (x+0.25)^2#
#x^2+0.5x-3 = (x+0.25)^2-3.0625#
#6((x+0.25^2)-3.0625) = 6(x+0.25^2) - 18.375#
#a(x-h)^2 + k = 6(x+0.25)^2 - 18.375#
turning point: #(-h,k)#, where #x=-h# is the axis of symmetry.
#(-h, k) = (-0.25,-18.375)#
#x= -0.25# is the axis of symmetry.
since the coefficient of #x^2# is positive #(6)#, the graph opens to the top.
#(-h, k)#, therefore, is a minimum point.
#(-h, k) = (-0.25, -18.375)#
since the minimum point is the lowest possible, the range is equal to or above #-18.375#.
#{y:y>=-18.375}#




