A monopolist faces a demand curve P = 70 - 1Q, with marginal revenue MR = 70 - 2Q, and MC = 20. Price is expressed in dollars.?
a)How to graph the three functions on one diagram.
b) How to compute the profit-maximizing output and price combination on the graph.
c) How to compute the efficient level of output (where MC = demand) on the graph
d) How to compute the deadweight loss associated with producing the profit-maximizing output rather than the efficient output ?
a)How to graph the three functions on one diagram.
b) How to compute the profit-maximizing output and price combination on the graph.
c) How to compute the efficient level of output (where MC = demand) on the graph
d) How to compute the deadweight loss associated with producing the profit-maximizing output rather than the efficient output ?
1 Answer
Profit maximising quantity and price combination.
Price
Quantity
DWL
Explanation:
Given -
#p=70 - Q# ------ -----------[Demand function]
#MR=70-2Q# ---------------[Marginal Revenue function]
#MC=20# --------------------[Marginal Cost function]
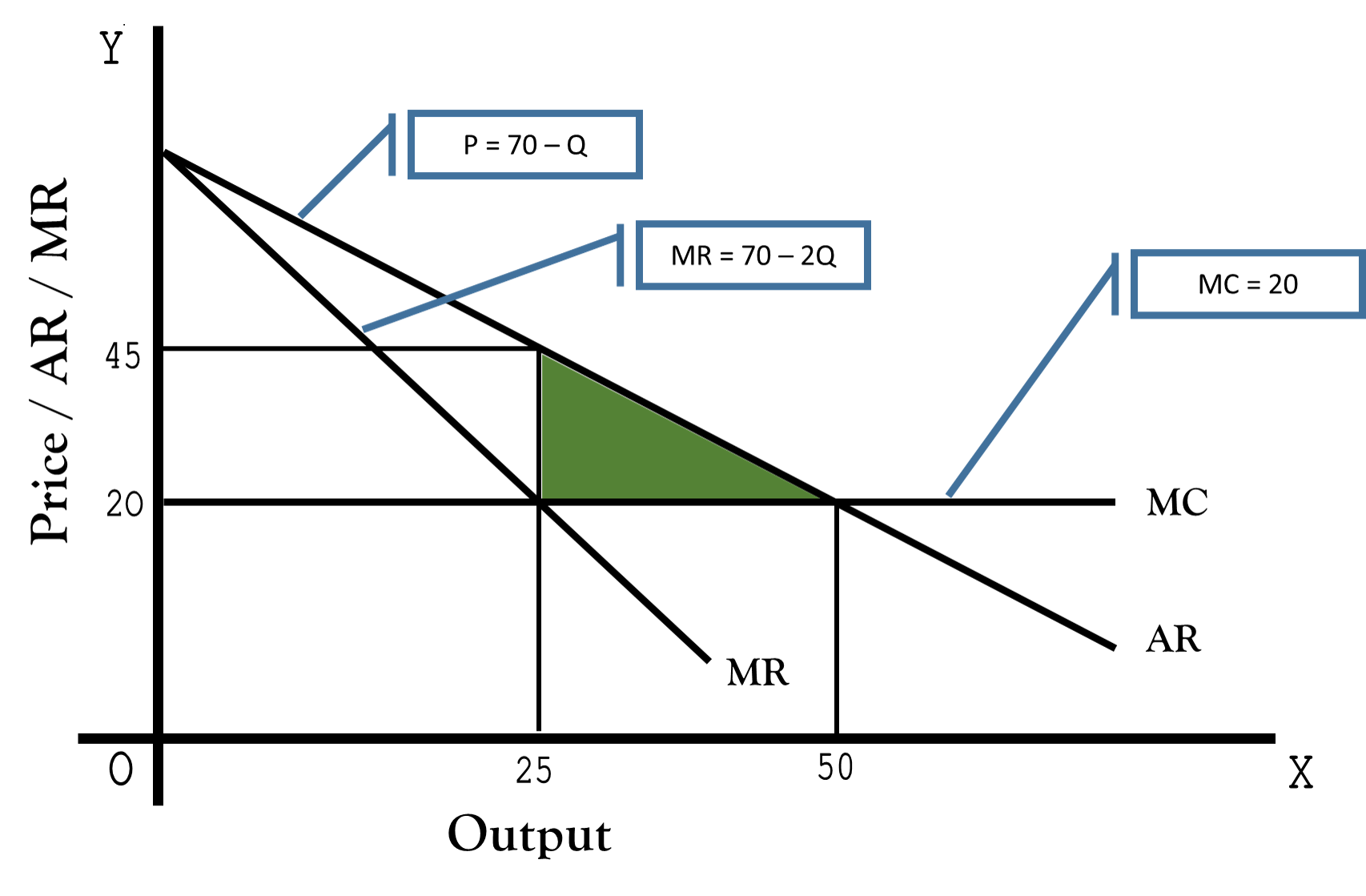
Profit Maximising Price and Output combination
Condition for maximum profit
#MR = MC#
#70-2Q=20#
#-2Q=20-70=-50#
#Q=(-50)/(-2)=25#
Profit maximising quantity#=25# units
Substitute
#p=70-Q#
#p=70-25=45#
Profit maximising price
Condition for Efficient level of output
#D= MC#
#70-Q=20#
#-Q=20-70=50#
#Q=50#
Efficient level of output
Dead Weight Loss is the green colour area.
DWL
DWL

