I was taught that if the adjacent length was longer than the opposite length of a known angle, there would be an ambiguous case of the sine rule. So why does d) and f) not have 2 different answers?


Any help would be appreciated, thanks!
All the best!


Any help would be appreciated, thanks!
All the best!
1 Answer
See below.
Explanation:
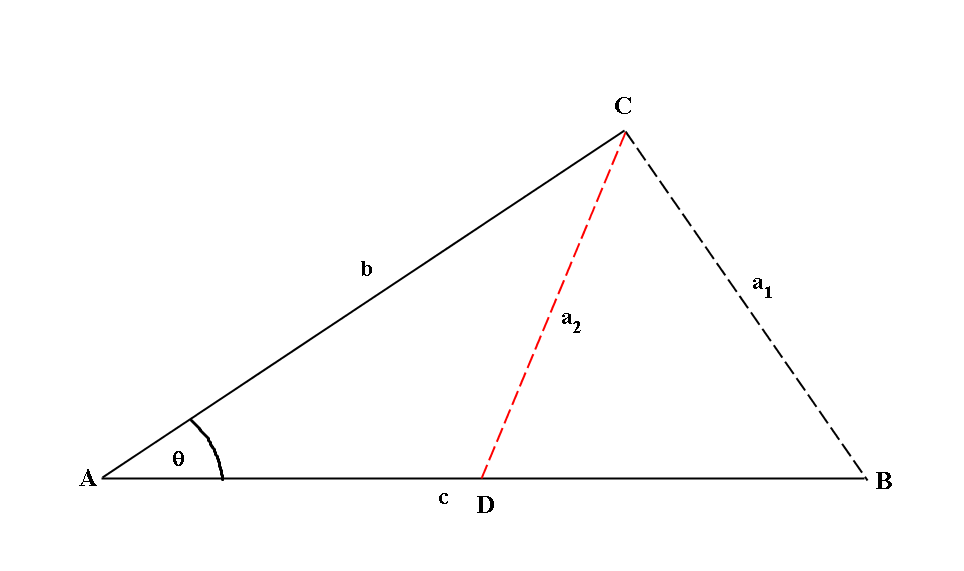
From the diagram.
i.e.
Suppose we are given the following information about the triangle:
Now suppose we want to find the angle at
Using the Sine Rule:
Now the problem we face is this.
Since:
Will we be calculating angle
As you can see, both these triangle fit the criteria we were given.
The ambiguous case will most likely occur when we are given one angle and two sides, but the angle is not between the two given sides.
You say you were told that if the adjacent side is longer than the opposite side then it would be an ambiguous case. This is not true:
Looking again at the diagram.
In triangle
If we are given the angle at
The side
The side
This dose not lead to the ambiguous case because, with some thought we can see that if
This is the case for your questions (d) and (f)
questions (b) and (c) are the same case I used in the diagram.
Explaining this is incredibly difficult. The best way to understand how altering angles and sides is with the use of interactive graphics. If you go online there are some sites where you can manipulate a triangle and see what the results of doing this are.
I hope I haven't confused you more.

