If a projectile is shot at a velocity of #1 ms^-1# and an angle of #pi/6#, how far will the projectile travel before landing?
1 Answer
Explanation:
For Physics or Mechanics you should learn the "suvat" equations for motion under constant acceleration:
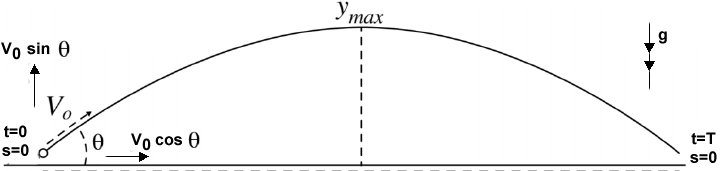
Vertical Motion
Motion under constant acceleration due to gravity, applied vertically upwards
Let the total time that the projectile is in the air be
# { (s=,0,m),(u=,(1)sin(pi/6)=1/2,ms^-1),(v=,"not required",ms^-1),(a=,-g,ms^-2),(t=,T,s) :} #
So we can calculate
# :. 0=1/2T-1/2gT^2 #
# :. 1/2T(1-gT)=0 #
# :. T=0, 1/g#
Horizontal Motion
Under constant speed (NB we can still use "suvat" equation with a=0). Thje projectile will be in in the air for the same time,
# s=(1)cos(pi/6)T #
# :. s=sqrt(3)/2T #
# :. s=sqrt(3)/2*1/g #
So using
#s=sqrt(3)/19.6 = 0.0883699...= 0.088 m# , or#8.8cm#

