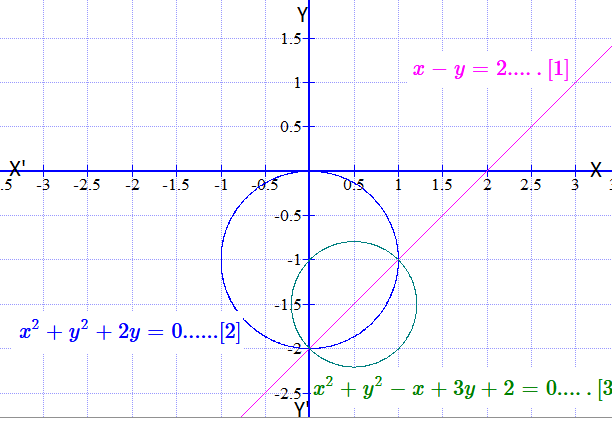
If #x-y=2# is the equation of a chord of the circle #x^2+y^2+2y=0#.Find the equation of the circle of which this chord is a diameter.?

2 Answers
P dilip_k
Nov 9, 2017

drawn

Given that

  • the equation of the chord #" "color (magenta)(x-y=2.....[1])#

  • the equation of the circle #" "color(blue)(x^2+y^2+2y=0......[2])#

From these two equations we get

#(y+2)^2+y^2+2y=0#

#=>2y^2+6y+4=0#

#=>2y^2+6y+4=0#

#=>y^2+3y+2=0#

#=>y^2+2y+y+2=0#

#=>y(y+2)+1(y+2)=0#

#=>(y+2)(y+1)=0#

So #y=-1 and-2#

Inserting in [1] we get

#x= 1 and 0# respectively

So the coordinates of points intersections of the chord with the circles are #(1,-1) and (0,-2)#
The line segment of the chord is also the diameter of a circle.

So equation of this circle having diameter #x-y=2# will be

#(y+1)/(x-1)xx(y+2)/(x-0)=-1#

#=>(y+1)xx(y+2)=-x(x-1)#

#color(green)(=>x^2+y^2-x+3y+2=0.....[3])#

This is the required equation of the circle

Ratnaker Mehta
Nov 9, 2017

# x^2+y^2-x+3y+2=0,#

Explanation:

We will solve this Problem using the following Result R :

R : The Equation (eqn.) of a Circle that passes through

the Points (pt.) of Intersection of a Circle S & Line L

#S : x^2+y^2+2gx+2fy+c=0 and L : lx+my+n=0,# is

#S+lambdaL : x^2+y^2+2gx+2fy+c+lambda(lx+my+n)=0,#

where, #lambda in RR.#

Let, #S : x^2+y^2+2y=0, and L : x-y-2=0.#

Note that, the Reqd. Circle, say #S',# passes through the pts. of

#SnnL.#

Applying R, we may suppose that, #S' : S+lambdaL=0, i.e., #

#S' : x^2+y^2+2y+lambda(x-y-2)=0.#

#:. S' : x^2+lambdax+y^2+(2-lambda)y=2lambda.#

#:. S' : x^2+lambdax+lambda^2/4+y^2+(2-lambda)y+(2-lambda)^2/4=2lamda+lambda^2/4+(2-lambda)^2/4, or,#

#S': (x+lambda/2)^2+(y+(2-lambda)/2)^2=2lamda+lambda^2/4+(2-lambda)^2/4.#

This shows that the Centre #C# of #S'# is #C(-lambda/2,-(2-lambda)/2).#

Now, given that #L# is a diameter of #S' rArr C in L.#

#rArr -lambda/2-(-(2-lambda)/2)=2.#

#:. -lambda/2+(2-lambda)/2=2, or, -lambda=2-1=1, i.e., lambda=-1.#

Therefore, #S': x^2+y^2+2y-1(x-y-2)=0, i.e.,#

# S' : x^2+y^2-x+3y+2=0,# is the reqd. eqn. of the Circle, as

already derived by Respected dk_ch Sir!

Enjoy Maths.!

Related questions
Impact of this question
9697 views around the world
You can reuse this answer
Creative Commons License