Question

In a triangle ABC, AB=AC and D is a point on side AC such that BCxBC=ACxCD. How to prove that BD=BC?

Answer 1

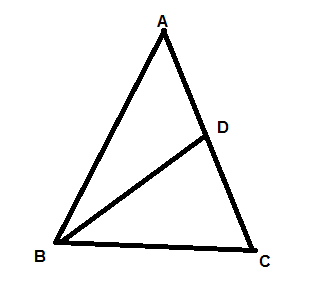
Given

`"In "DeltaABC`

`AB=AC and D" is a point on"AC " such that"`

`BCxxBC=ACxxAD`

`"We are to prove "BD=BC`

Proof

Rearrenging the given relation

`BCxxBC=ACxxAD" "` We can write

`(BC)/(CD)=(AC)/(BC)->DeltaABC" similar "DeltaBDC`

Their corresponding angle pairs are:

`1. /_BAC "= corresponding "/_DBC`

`2. /_ABC "= corresponding "/_BDC`

`3. /_ACB " =corresponding "/_DCB`

So as per above relation 2 we have
`/_ABC =" corresponding "/_BDC`

`"Again in"DeltaABC`

`AB=AC->/_ABC=/_ACB=/_DCB`

`:."In "DeltaBDC,/_BDC=/_BCD`

`->BD=BC`

Alternative way

The ratio of corresponding sides may be written in extended way as follows

`(BC)/(CD)=(AC)/(BC)=(AB)/(BD)`

From this relation we have

`(AC)/(BC)=(AB)/(BD)`

`=>(AC)/(BC)=(AC)/(BD)->"As "AB=AC" given"`

`=>1/(BC)=1/(BD)`

`=>BC=BD`

Proved

Hope, this will help