Question

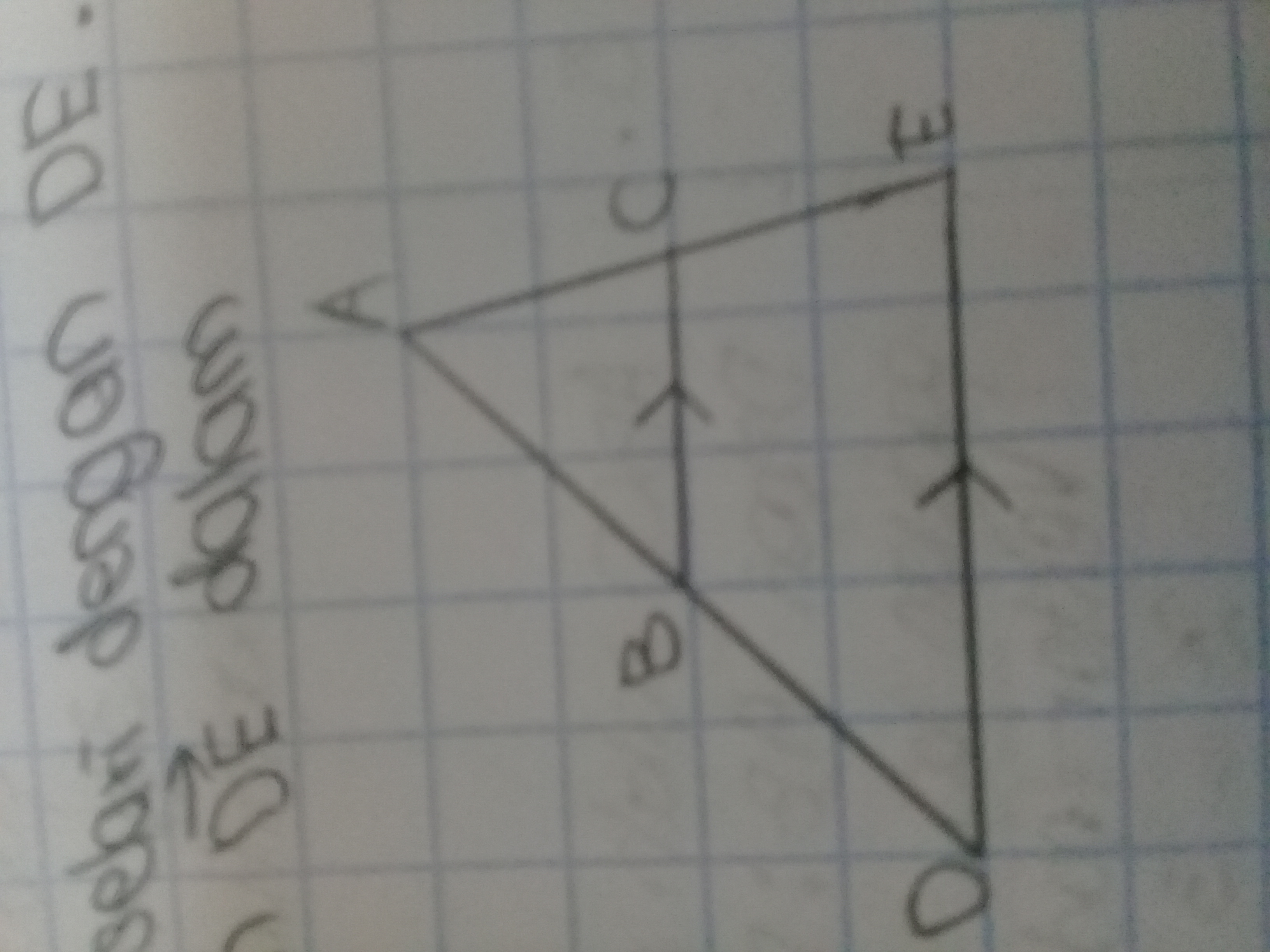
In the below diagram, BC is parallel to DE. Given AC:CE=2:3, express `vecDE` in term of `vecBC`?

Answer 1

`DE = 5/2BC`

Explanation:

If BC is parallel to DE, then the triangles are similar, which means that all sides are proportional.

We are given that AC:CE = 2:3

So, the ratio of AC to the comparable side of the larger triangle, which is AE = AC + CE is:

`2/(2+3) = 2/5`

So, they are similar triangles, so BC/DE is the same ratio:

`(BC)/(DE) = 2/5`

...you can cross multiply:

`5BC = 2DE`

...and then you can write an equation for DE in terms of BC:

`DE = 5/2BC`

GOOD LUCK