Integration of sinx/x from 0 to infinity?
1 Answer
# int_0^oo \ sinx/x \ dx = pi/2#
Explanation:
We seek:
# I = int_0^oo \ sinx/x \ dx #
Let
Thus
# 2I = int_(-oo)^oo \ sinx/x \ dx #
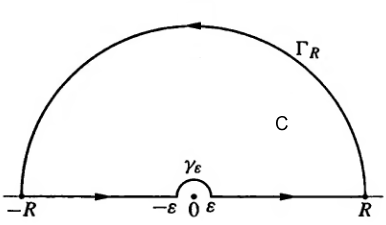
Consider the complex based function
# J = oint_C \ f(z) \ dz = oint_C \ e^(iz)/z \ dz # where#z in CC#
Where
The integrand has no poles in
# oint_C \ f(z) \ dz = 0#
Now (in shorthand),
# oint_C \ f(z) \ dz = int_(-R)^(epsilon) + int_(gamma_epsilon) + int_(epsilon)^R + int_(Gamma_R) = 0#
We require an estimate for
# abs(int_(Gamma_R) \ e^(iz)/z \ dz) = abs(int_o^oo \ e^(iRcos theta-R sin theta) / (Re^(i theta)) iRe^(i theta) \ d theta ) #
# " " le int_0^pi e^(-Rsin theta) \ d theta #
# " " = 2 int_0^(pi/2) e^(-Rsin theta) \ d theta #
# " " le 2 int_0^(pi/2) e^((-2R theta) / pi) \ d theta \ \ \ \# using#sin theta ge (2theta)/pi#
# " " = 2 [ (2e^((-2Rtheta)/pi) )/ ((-2R)/pi) ]_0^(pi/2)#
# " " = pi/R(1-e^-R)#
# " " rarr 0 # as#R rarr oo#
Given that the small circle
# lim_(epsilon rarr 0) int_(gamma_epsilon) \ f(z) \ dz = i \ lim_(epsilon rarr 0) int_pi^0 \ e^(-rsin theta)e^(ircos theta) \ dz #
# " " = -pi i #
Taking the two limits
# int_(-oo)^oo e^(iz)/z \ dz - pi i = 0 => int_(-oo)^oo (cosx+isinx)/x \ dx = pi i #
Equating real and imaginary coefficients we get:
# Re: int_(-oo)^oo cosx/x \ dx = 0 #
# Im: int_(-oo)^oo sinx/x \ dx = pi #
Then using the initial result
# 2I = int_(-oo)^oo \ sinx/x \ dx => 2I = pi#
Hence,
# I = pi/2 #