Kindly solve these 2 questions?
(a) If a circle is inscribed in a right angled triangle ABC with the right angle at B, show the diameter of the circle is equal to AB + BC – AC.
(b) If a triangle is inscribed in a circle, then the product of any two sides of the triangle is equal to the product of the diameter and the perpendicular distance of the third side from the opposite vertex. Prove the above statement.
(a) If a circle is inscribed in a right angled triangle ABC with the right angle at B, show the diameter of the circle is equal to AB + BC – AC.
(b) If a triangle is inscribed in a circle, then the product of any two sides of the triangle is equal to the product of the diameter and the perpendicular distance of the third side from the opposite vertex. Prove the above statement.
1 Answer
(a)
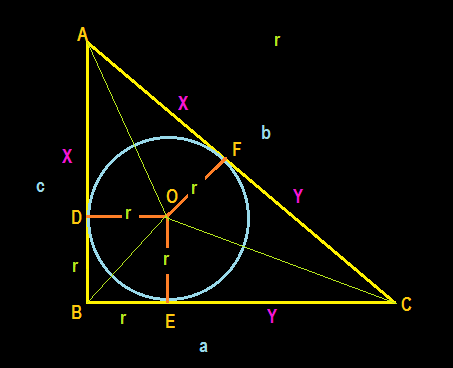
O is the center of in-circle of the right angled triangle
O being the in-center of the
So
So
So
Again
Hence
Now
(b)
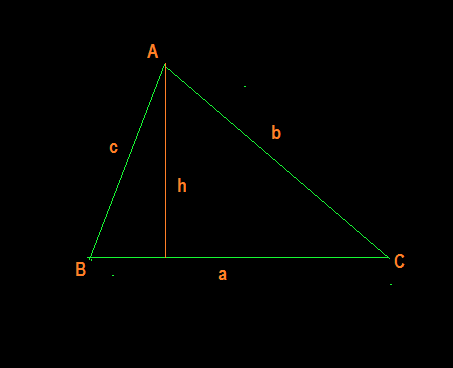
By sine law we have for
where R is the radius of the circumcircle of
Now from relation [1] we have
where h is the length of the perpendicular from A to BC
So
This relation can be proved similarly for any pair of sides of
So for a triangle inscribed in a circle, the product of any two sides of the triangle is equal to the product of the diameter and the perpendicular distance of the third side from the opposite vertex

