Momentum ?

Question

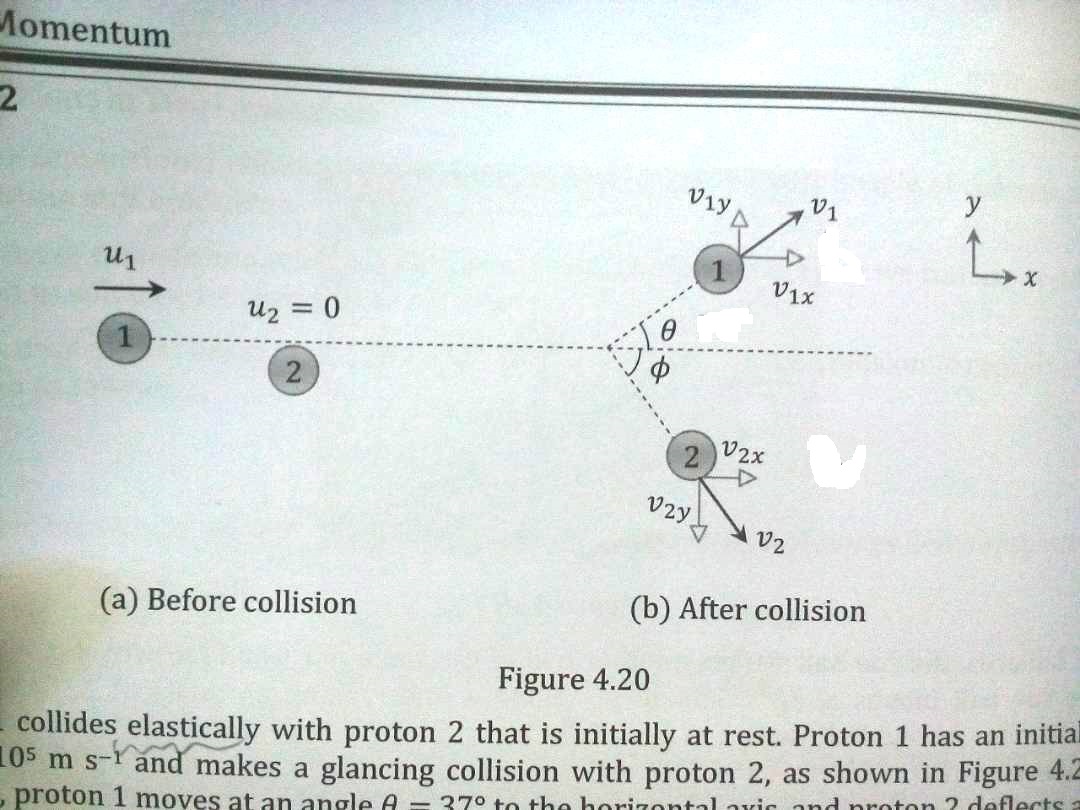
Proton 1 collides elastically with proton 2 that is initially at rest. Proton 1 has an initial speed #u_1=3.5010^5ms^-1# and makes a glancing collision with proton 2, as shown in Figure 4.20. After the collision, proton 1 moves at an angle #theta=37^@# to the horizontal axis, and proton 2 deflects at an angle #phi# with to the same axis. Find the final speeds of the two protons and the angle #phi#.

The answer provided are#[2.8010^5ms^-1, 2.11*10^5ms^-1, 53^@]#, but i want to know how to solve.

2616 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-09-24T04:19:42

See below.

#m_1 vec v_0 = m_1 vec v_1 + m_2 vec v_2#

#vec v_0 = v_0(1,0)#
#vec v_1 =v_1 (cos theta,sin theta)#
#vec v_2 =v_2 (cos phi,-sin phi)#

then

#{(v_0=v_1costheta+v_2cosphi),(0=v_1sintheta-v_2sinphi):}#

and solving for #v_1,v_2#

#{(v_1 =(v_0 Sin phi)/(Cos theta Sin phi + Cos phi Sin theta) ),(v_2 =(v_0 Sin theta)/(Cos theta Sin phi + Cos phi Sin theta)):}#

Now the collision is perfectly elastic so

#1/2m_1 v_0^2=1/2m_1 v_1^2+1/2m_2 v_2^2#

or

#((v_0^2Sin theta)/(sin(phi+theta)^2) )(m_1 Sin(2 phi + theta)-m_2 Sin theta)=0#

then from

#(m_1 Sin(2 phi + theta)-m_2 Sin theta)=0#

with #m_1 = m_2# we easily determine #phi# and consequently #v_1# and #v_2#