Question

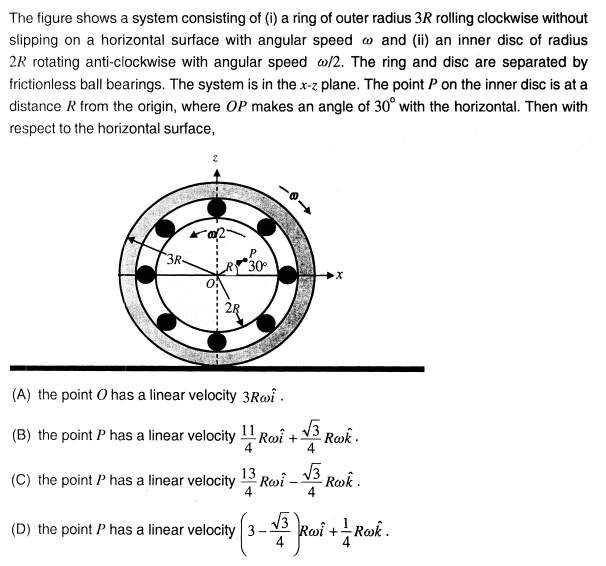
Please give the correct options with proper explanation for each correct options?

Answer 1

(A) and (B)

Explanation:

As the outer ring rolls without slipping therefore any point on the ring has linear velocity given by the equation for circular motion

`v=romega`

Point `O` is also the centre of mass of the ring

`:.|vecV_(cm)|=|vecV_O|=(3R)ω`

Because the ring rolls clockwise, from the figure velocity vector has the direction of `hati`
`:.vecV_(cm)=vecV_O=3Rωhat i`

Linear velocity of point `P` has two components.
1. `vecV_(cm)`
2. `vecV_(P_"disk cm")`

Point `P` as a part of Disk has magnitude of linear velocity `=Romega/2`
To ascertain direction of this component see figure below. We need to state unit vector in the direction of tangent at `P` and its `xand z` components as shown.

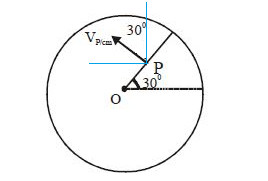
Total linear velocity of point `P`
`vec V_P=\vec V_(cm)+\vec V_(P_("disk cm"))=3R\omega\hat i+{\omega R}/{2}(cos30\hat k-sin30\hat i)`
`=>vec V_P=3R\omega\hat i+{omega R}/{2}(sqrt3/2hat k-1/2hat i)`
`=>vec V_P={11}/{4}Romegahat i+{sqrt3}/{4}Romega hat k`