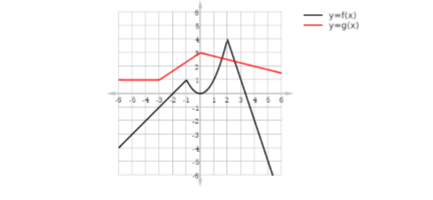
Suppose #h(x) = f(x)g(x)# Use the above graph determine if h is increasing, decreasing or neither at #x = 4# , #x=1#, and #x=-5#? (See image)

enter image source here

1 Answer
marfre
Mar 18, 2017

Decreasing at #x=4#
Increasing at #x=1#
Increasing at #x=-5#

Explanation:

The function #h(x) = f(x)g(x)# is just the multiplication of the y-values given an x-value for each function.

Since #f(x) = 1# for the interval #(-oo, -3)#, #h(x) = f(x)# for that interval.
#h(-1) = f(-1)*g(-1) = 1*g(-1)#
#h(0) = f(0)*g(0) = 0#
#h(2) = f(2)*g(2) = 4(2.5) = 10#
#h(3) = f(3)*g(3) = 1*g(3) =g(3)#
#h(3.5) = f(3.5)*g(3.5) = 0*g(3.5) = 0#
#h(5) = f(5)*g(5) = -5*1.75 = -8.75#

#h(x)# is increasing from #(-oo, -1), (0,2)#
#h(x)# is decreasing from #(-1, 0), (2, oo)#

enter image source here

Related questions
Impact of this question
2056 views around the world
You can reuse this answer
Creative Commons License