Question

The number of square meters in the total surface area of a right circular cylinder, including the top and bottom, is equal to the number of cubic meters in its volume. If the radius of the cylinder is five times its height, what is its volume?

Answer 1

See a solution process below:

Explanation:

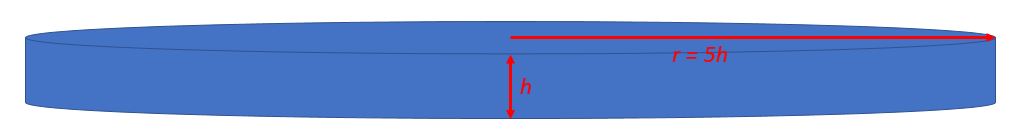
We know `r = 5h` for the cylinder

The formula for the volume of a cylinder is:

`V = pir^2h`

We can substitute `5h` for `r` giving:

`V = pi(5h)^2h`

`V = pi25h^2h`

`V = pi25h^3`

`V = 25pih^3`

The formula for the surface area of a cylinder is:

`A = 2pirh + 2pir^2`

Again, we can substitute `5h` for `r` giving:

`A = (2pi5h xx h) + 2pi(5h)^2`

`A = 10pih^2 + 2pi25h^2`

`A = 10pih^2 + 50pih^2`

`A = (10 + 50)pih^2`

`A = 60pih^2`

Because the Area is equal to the volume we can equate the two and solve for `h`:

`60pih^2 = 25pih^3`

`(60pih^2)/(25pih^2) = (25pih^3)/(25pih^2)`

`(60color(red)(cancel(color(black)(pih^2))))/(25color(red)(cancel(color(black)(pih^2)))) = (color(red)(cancel(color(black)(25pi)))h^3)/(color(red)(cancel(color(black)(25pi)))h^2)`

`60/25 = h^3/h^2`

`(5 xx 12)/(5 xx 5) = (h^2 xx h)/h^2`

`(color(red)(cancel(color(black)(5))) xx 12)/(color(red)(cancel(color(black)(5))) xx 5) = (color(red)(cancel(color(black)(h^2))) xx h)/color(red)(cancel(color(black)(h^2)))`

`12/5 = h`

`h = 12/5`

The we can calculate `r` as `r = 5 xx 12/5 = 12`

We can substitute these back into the formula for the volume of a cylinder and calculate `V`:

`V = pir^2h` becomes:

`V = pi xx 12^2 xx 12/5`

`V = pi xx 144 xx 12/5`

`V = pi xx 1728/5`

`V = pi xx 345.6`

`V = 345.6pi`

Approximating `pi` with 3.14 gives:

`V = 345.6pi ~= 345.6 xx 3.14 ~= 1085.184`