Question

The radii of two concentric circles are 16 cm and 10 cm. `AB` is a diameter of the bigger circle. `BD` is tangent to the smaller circle touching it at `D`. What is the length of `AD`?

Answer 1

`bar(AD)=23.5797`

Explanation:

Adopting the origin `(0,0)` as the common center for `C_i` and `C_e` and calling `r_i=10` and `r_e=16` the tangency point `p_0=(x_0,y_0)` is at the intersection `C_i nn C_0` where

`C_i->x^2+y^2=r_i^2`
`C_e->x^2+y^2=r_e^2`
`C_0->(x-r_e)^2+y^2= r_0^2`

here `r_0^2 = r_e^2-r_i^2`

Solving for `C_i nn C_0` we have

`{(x^2+y^2=r_i^2),((x-r_e)^2+y^2=r_e^2-r_i^2):}`

Subtracting the first from the second equation

`-2xr_e+r_e^2=r_e^2-r_i^2-r_i^2` so

`x_0 = r_i^2/r_e` and `y_0^2= r_i^2-x_0^2`

Finally the sought distance is

`bar(AD)=sqrt((r_e+x_0)^2+y_0^2)=sqrt(r_e^2+3r_i^2)`

or

`bar(AD)=23.5797`

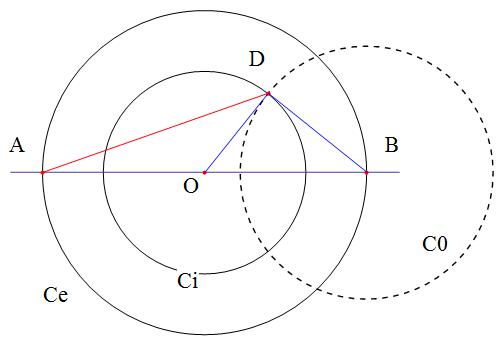
Explanation:

If `bar(BD)` is tangent to `C_i` then `hat(ODB) = pi/2` so we can apply pythagoras:

`bar(OD)^2+bar(DB)^2=bar(OB)^2` determining `r_0`

`r_0^2= bar(OB)^2-bar(OD)^2=r_e^2-r_i^2`

The point `D` coordinates, called `(x_0,y_0)` should be obtained before calculating the sought distance `bar(AD)`

There are many ways to do that. An alternative method is

`y_0=bar(BD)sin(hat(OBD))` but `sin(hat(OBD))=bar(OD)/bar(OB)`

then

`y_0 = sqrt(r_e^2-r_i^2)(r_i/r_e)` and
`x_0=sqrt(r_i^2-y_0^2)`

Answer 2

As per given data the above figure is drawn.

O is the common center of two concentric circles

`AB->"diameter of the bigger circle"`

`AO=OB->"radius of the bigger circle"=16 cm`

`DO->"radius of the smaller circle"=10cm`

`BD->"tangent to the smaller circle"->/_BDO=90^@`

Let `/_DOB=theta=>/_AOD=(180-theta)`

In `Delta BDO->cos/_BOD=costheta=(OD)/(OB)=10/16`

Applying cosine law in `Delta ADO` we get

`AD^2=AO^2+DO^2-2AO*DOcos/_AOD`

`=>AD^2=AO^2+DO^2-2AO*DOcos(180-theta)`

`=>AD^2=AO^2+DO^2+2AO*DOcostheta`

`=>AD^2=AO^2+DO^2+2AO*DOxx(OD)/(OB)`

`=>AD^2=16^2+10^2+2xx16xx10xx10/16`

`=>AD^2=556`

`=>AD=sqrt556=23.58cm`