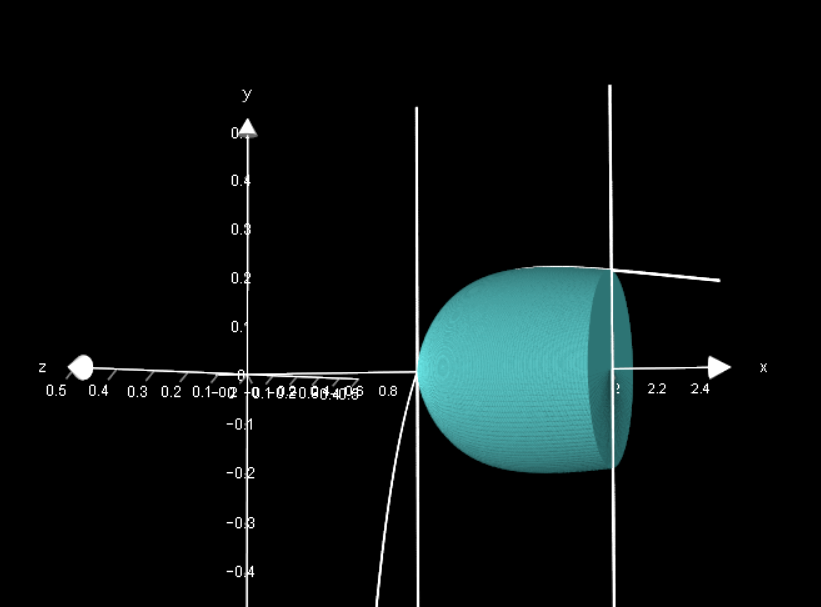
The region under the curve #y=lnx/x^2, 1<=x<=2# is rotated about the x axis. How do you find the volume of the solid of revolution?
1 Answer
See below.
Explanation:
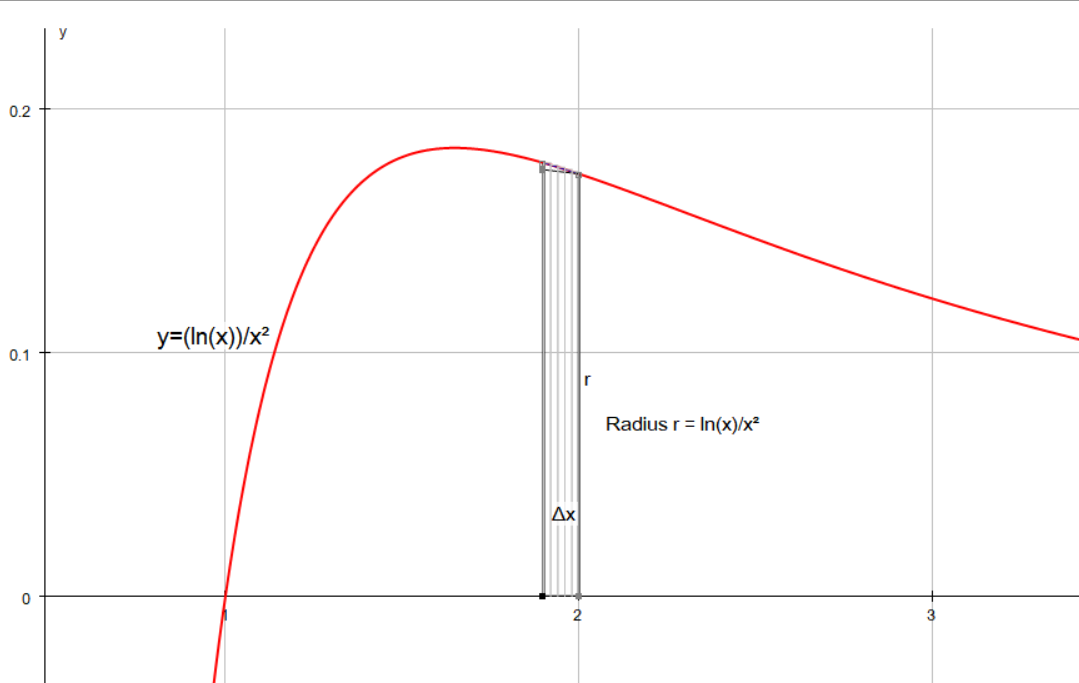
To find the volume of revolution, we use the idea of a series of discs. We find the volume of each disc and these are summed together. From the diagram, we can see that the rectangle has a height of
We then sum all the disc in the interval together to find the total volume of the solid. This is the same idea as when we find the area between a curve and the axes.
Plugging in the upper and lower bounds:
Volume of revolution: