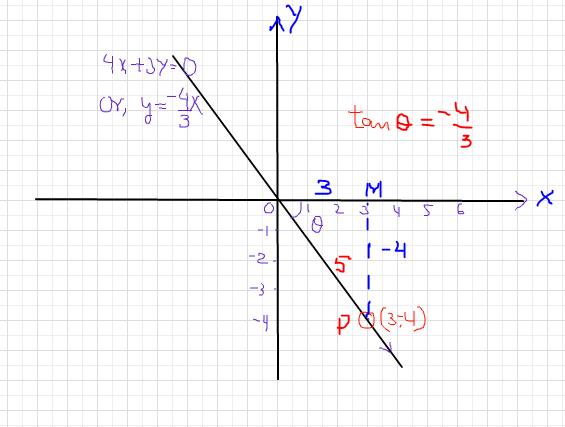
The terminal side of #theta# lies on the line #4x+3y=0# in quadrant IV, how do you find the values of the six trigonometric functions by finding a point on the line?
1 Answer
Explanation:
The slope of the given line is
The angle
As shown in the figure below, triangle OPM is a rt. triangle, its hypotenuse is 5 with base and altitude being 3 and -4 respectively. Accordingly,