What are scientific models?
1 Answer
Scientific models are objects or concepts constructed to explain phenomena that may not be technically observable.
Even in higher levels of chemistry, models are very useful, and are often constructed to estimate chemical properties. An example below illustrates the use of models to estimate a known quantity.
Suppose we want to model benzene,
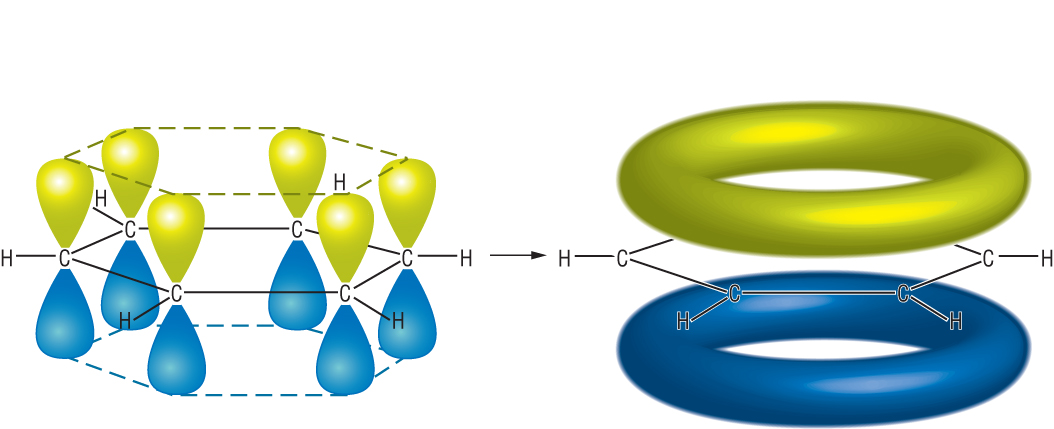
The true value is
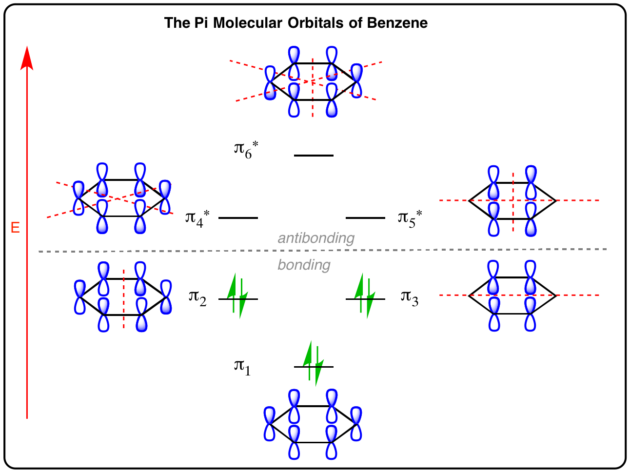
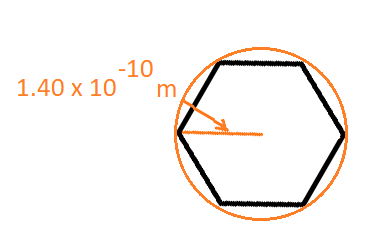
MODEL 1: PARTICLE ON A RING
The Particle on a Ring model is useful for describing the
The energy levels are:
#E_k = (ℏ^2k^2)/(2I)# ,#" "k = 0, pm1, pm2, . . . # where:
#I = m_eR^2# is the moment of inertia for the particle as a point mass a constant radial distance#R# away from#O# .#k = sqrt((2IE)/ℏ^2)# is the quantum number for this system.#ℏ = (6.626 xx 10^(-34) "J"cdot"s")/(2pi)# is the reduced Planck's constant.#m_e = 9.109 xx 10^(-31) "kg"# is the mass if an electron is the particle.#c = 2.998 xx 10^8"m/s"# , the speed of light, will be needed.
The strongest electronic transition corresponds to
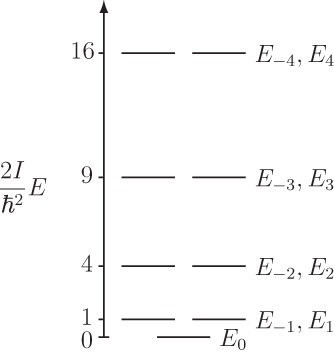
If we use this knowledge, we can estimate the wavelength observed for the strongest electronic transition. It is known experimentally that
The energy gap is:
#DeltaE_(1->2) = ℏ^2/(2I)(2^2 - 1^2)#
From the relation that
#color(blue)(lambda) = (hc)/(DeltaE) ~~ (hc)/(DeltaE_k) = (hc cdot 2m_eR^2)/(ℏ^2(2^2 - 1^2))#
#= (4pi^2 cdot hc cdot 2m_eR^2)/(3h^2)#
#= (8pi^2 cm_eR^2)/(3h)#
#= (8pi^2 cdot 2.998 xx 10^8 "m/s" cdot 9.109 xx 10^(-31) "kg" cdot (1.40 xx 10^(-10) "m")^2)/(3(6.626 xx 10^(-34) "J"cdot"s"))#
#= 2.13 xx 10^(-7) "m"#
#=# #color(blue)("213 nm")#
MODEL 2: PARTICLE IN A BOX
The Particle in a Box model can also be used for the same purpose. We can confine benzene into a
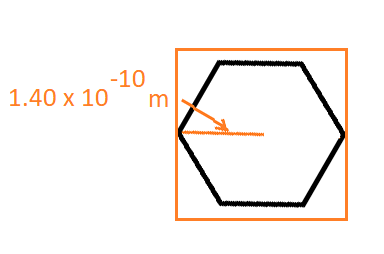
In two dimensions, the energy levels are:
#E_(n_xn_y) = (h^2)/(8m_e)[n_x^2/L_x^2 + n_y^2/L_y^2]# ,
#n_x = 1, 2, 3, . . . #
#n_y = 1, 2, 3, . . . #
The first few are:
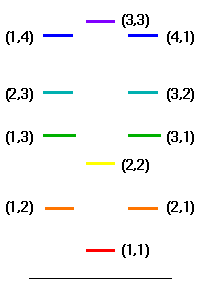
which matches the way the energy levels are in benzene exactly, if we call
#DeltaE_(12 -> 13) = (h^2)/(8m_e)[(cancel(1^2/L_x^2) + 3^2/L_y^2) - (cancel(1^2/L_x^2) + 2^2/L_y^2)]#
#= (h^2)/(8m_e)((3^2 - 2^2)/L_y^2)#
#= (6.626 xx 10^(-34)"J"cdot"s")^2/(8cdot9.109 xx 10^(-31) "kg")((3^2 - 2^2)/(2.80 xx 10^(-10) "m")^2)#
#= 3.84 xx 10^(-18) "J"#
And so, the wavelength involved is estimated to be:
#color(blue)(lambda) = (hc)/(DeltaE_(12->13)) = (6.626 xx 10^(-34) "J"cdot"s" cdot 2.998 xx 10^8 "m/s")/(3.84 xx 10^(-18) "J")#
#= 5.17 xx 10^(-8) "m"#
#=# #color(blue)"51.7 nm"#
So as it turns out, the particle on a ring is more effective of a model for benzene.

